-
Chapter 14: GEOMETRY
Introduction
- Sum of the angles of a triangle is 180°.
- Sum of any two sides of a triangle is greater than the third side.
- Area of a rectangle = length * breadth.
- Area of a square = side^2 = 1/2(diagonal)^2
- Area of a circle = π * r*r ; r = radius and π / PI = 22/7 or 3.14
- Area of a kite: 1/2 * (sum of the length of two diagonals)
- Area of a semi circle = π * r * r / 2 ; Circumference = π * r
- To find the area of a parallelogram, just multiply the base length (b) times the height (h): Area = b * h
Volume and Surface Area
Cuboid: If length = L, breadth = B, height = H then Volume = L * B * H.
volume of a cube = a3 , Surface area = 6 *a * a sq m, diagonal = sq-root(3) * a
cylinder = b h = π * r2 h
pyramid = (1/3) b h
volume of a cone = (1/3) b h = 1/3 * π *r2 h
volume of a sphere = (4/3) * π * r3
Basic Conversions
1 m = 100 cm = 1000 mm
1 km = 1000 m = 5/8 miles
1 inch = 2.54 cm
100 kg = 1 quintal
10 quintal = 1 tonne = 1000 kg
1 kg = 2.2 pounds
1 m = 39.37 inches
1 mile = 1760 yd = 5280 ft; 1 nautical mile (knot) = 6080 ft
1 litre = 1000 cc
1 acre = 100 sq m; 1 hectare = 10000 sq m
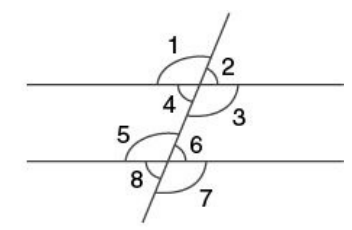
STRAIGHT LINES
Parallel Lines : Two straight lines are parallel if they lie on the same plane and do not intersect however far produced.
Transversal: It is a straight line that intersects two parallel lines. When a transversal intersects two parallel lines then
Corresponding angles are equal, (that is: For the following figure) 1 = 5; 2 = 6; 4 = 8; 3 = 7
Alternate interior angles are equal, that is (Refer following figure) 4 = 6; 5 = 3
Alternate exterior angles are equal, that is 2 = 8; 1 = 7
Interior angles on the same side of transversal add up to 180°, that is 4 + 5 = 3 + 6 = 180°
POLYGONS
Polygons are plane figures formed by a closed series of rectilinear (straight) segments. Example: Triangle, Rectangle
Regular polygons: Polygons with all the sides and angles equal.
Irregular polygons: Polygons in which all the sides or angles are not of the same measure.
Polygon can also be divided as concave or convex poly-gons. Convex polygons are the polygons in which all the diagonals lie inside the figure otherwise it’s a concave polygon
| No. of sides | Name of the polygon | Sum of all the angles |
|---|---|---|
| 3 | Triangle | 180 |
| 4 | Quadrilateral | 360 |
| 5 | Pentagon | 540 |
| 6 | Hexagon | 720 |
| 7 | Heptagon | 900 |
| 8 | Octagon | 1080 |
| 9 | Nonagon | 1260 |
| 10 | Decagon | 1440 |
Properties
Sum of all the angles of a polygon with n sides = (2n – 4)p/2 or (n – 2)p Radians = (n – 2) 180° degrees
Sum of all exterior angles = 360°, i.e. In the figure below: θ1 + θ2 + ... + θ6 = 360°, In general, θ1 + θ2 + ... + θn = 360°
No. of sides = 360°/exterior angle
Area = (ns2/4) × cot (180/n); where s = length of side, n = no. of sides.
Perimeter = n × s.

TRIANGLES (D)
A triangle is a polygon having three sides. Sum of all the angles of a triangle = 180°.
Acute angle triangle: Triangles with all three angles acute (less than 90°)
Obtuse angle triangle: Triangles with one of the angles obtuse (more than 90°). we cannot have more than one obtuse angle in a triangle.
Right angle triangle: Triangle with one of the angles equal to 90°.
Equilateral triangle: Triangle with all sides equal. All the angles in such a triangle measure 60°
Isosceles triangle: Triangle with two of its sides equal and consequently the angles opposite the equal sides are also equal.
Scalene Triangle: Triangle with none of the sides equal to any other side.
Properties (General)
Sum of the length of any two sides of a triangle has to be always greater than the third side.
Difference between the lengths of any two sides of a triangle has to be always lesser than the third side
Side opposite to the greatest angle will be the greatest and the side opposite to the smallest angle the smallest
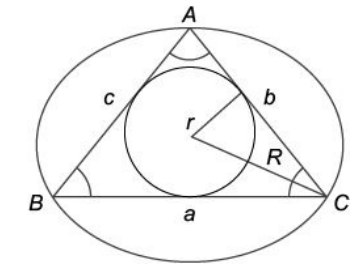
The sine rule: a/sin A = b/sin B = c/sin C = 2R (where R = circum radius.)
The cosine rule: a2 = b2 + c2 – 2bc cos A This is true for all sides and respective angles
In case of a right angle, the formula reduces to a2 = b2 + c2, Since cos 90 = 0
The exterior angle is equal to the sum of two interior angles not adjacent to it. –ACD = –BCE = –A + –B
Area = 1/2 base × height or 1/2 bh. Height = Perpendicular distance between the base and vertex opposite to it
Area = rs (where r is in radius)
Area = 1/2 × product of two sides × sine of the included angle
Area = \( \sqrt{s(s-a)(s-b)(s-c)} \) where \( s = \frac{a+b+c}{2}\)
= 1/2 ac sin B
= 1/2 ab sin C
= 1/2 bc sin A
Area = abc/4R; where R = circum radius


Congruency of Triangles
Two triangles are congruent if all the sides of one are equal to the corresponding sides of another. It follows that all the angles of one are equal to the corresponding angles of another. The notation for congruency is ≅
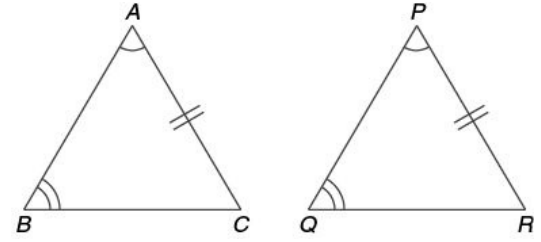
SAS congruency: If two sides and an included angle of one triangle are equal to two sides and an included angle of another, the two triangles are congruent. Here, AB = PQ, BC = QR, and ∠B = ∠Q ; So △ABC ≅ △PQR
ASA congruency: If two angles and the included side of one triangle is equal to two angles and the included side of another, the triangles are congruent. (See figure below.) Here, ∠A = ∠P, ∠B = ∠Q and AB = PQ , So △ABC ≅ △PQR
AAS congruency: If two angles and side opposite to one of the angles is equal to the corresponding angles and the side of another triangle, the triangles are congruent. ∠A = ∠P; ∠B = ∠Q; and AC = PR; So △ABC ≅ △PQR
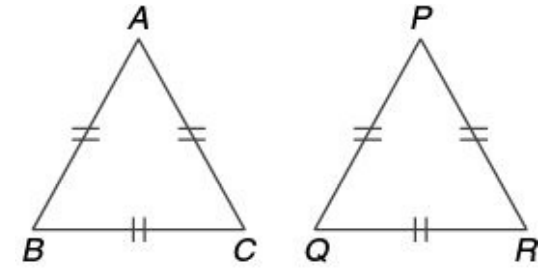
SSS congruency: If three sides of one triangle are equal to three sides of another triangle, the two triangles are congruent. AB = PQ, BC = QR, AC = PR, △ABC ≅ △PQR
SSA congruency: If two sides and the angle opposite the greater side of one triangle are equal to the two sides and the angle opposite to the greater side of another triangle, then the triangles are congruent. The congruency doesn’t hold if the equal angles lie opposite the shorter side. if AB = PQ; AC = PR; ∠B = ∠Q; Then the triangles are congruent. i.e. △ABC ≅ △PQR


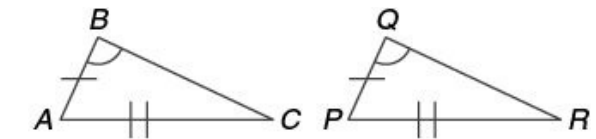
Similarity of triangles : Basic real life concept of similarity. i.e. Two things are similar if they look similar
Similarity of triangles is a special case where if either of the conditions of similarity of polygons holds, the other will hold automatically.
AAA similarity: If in two triangles, corresponding angles are equal, that is, the two triangles are equiangular then the triangles are similar.
Corollary (AA similarity): If two angles of one triangle are respectively equal to two angles of another triangle then the two triangles are similar. The reason being, the third angle becomes equal automatically
SSS similarity: If the corresponding sides of two triangles are proportional then they are similar. For DABC to be similar to DPQR, AB/PQ = BC/QR = AC/PR, must hold true
SAS similarity: If in two triangles, one pair of corresponding sides are proportional and the included angles are equal then the two triangles are similar. DABC ~ DPQR; If AB/BC = PQ/QR and ∠B = ∠Q
In similar triangles the following identity hold Ratio of medians = Ratio of heights = Ratio of circumradii = Ratio of inradii = Ratio of angle bisectors
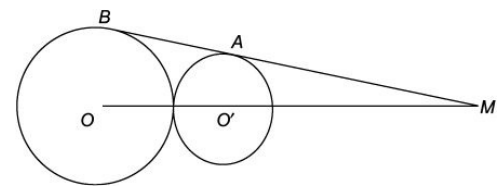
Thus, in the figure above if you were to draw the radii OB and OA the two triangles MOB and MOA will be similar to each other. Simply because they look similar. Of course, the option of using the different rules of similarity of triangles still remains with you.
Equilateral Triangles (of side a):
Area = 1/2 (base) × (height) = \( \frac{\sqrt{3} * a^2}{4} \). Height = \( \frac{\sqrt{3} * a}{2} \)
R (circum radius) = \( \frac{a}{\sqrt{3}} = \frac{2h}{3}\)
r (in radius) = \( \frac{a}{2\sqrt{3}} = \frac{h}{3}\)

Properties
The incentre and circumcentre lies at a point that divides the height in the ratio 2 : 1
The circum radius is always twice the in radius. [R = 2r.]
Among all the triangles that can be formed with a given perimeter, the equilateral triangle will have the maximum area.
An equilateral triangle in a circle will have the maximum area compared to other triangles inside the same circle
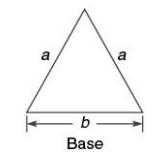
Isosceles Triangle : Area = \( \frac{b}{4} * \sqrt{4a^2 - b^2}\)
In an isosceles triangle, the angles opposite to the equal sides are equal
Right-Angled Triangle
Pythagoras Theorem In the case of a right angled triangle, the square of the hypotenuse is equal to the sum of the squares of the other two sides. In the figure below, for triangle ABC, a2 = b2 + c2 Area = 1/2 (product of perpendicular sides)
R(circumradius) = hypotenuse / 2
Area = rs (where r = in radius and s = (a + b + c)/2 where a, b and c are sides of the triangle)
1/2 bc = r(a + b + c)/2 ; r = (bc)/(a + b + c)
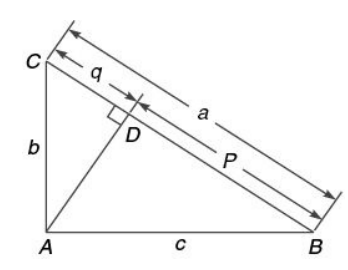
In the triangle ABC, △ABC ~ △DBA ~ △DAC
△ABC ~ △DBA; AB/BC = DB/BA; AB2 = DB × BC; c2 = pa
△ABC ~ △DAC; AC/BC = DC/AC; AC2 = DC × BC; b2 = qa
△DBA ~ △DAC; DA/DB = DC/DA; DA2 = DB × DC; AD2 = pq
Basic Pythagorean Triplets
3, 4, 5 ; 5, 12, 13 ; 7, 24, 25 ; 8, 15, 17 ; 9, 40, 41; 11, 60, 61 ; 12, 35, 37 ; 16, 63, 65 ; 20, 21, 29 ; 28, 45, 53. These triplets are very important since a lot of questions are based on them
Any triplet formed by either multiplying or dividing one of the basic triplets by any positive real number will be another Pythagorean triplet. Thus, since 3, 4, 5 form a triplet so also will 6, 8 and 10 as also 3.3, 4.4 and 5.5
Similarity of right triangles : Two right triangles are similar if the hypotenuse and side of one is proportional to hypotenuse and side of another. (RHS–similarity–Right angle hypotenuse side)
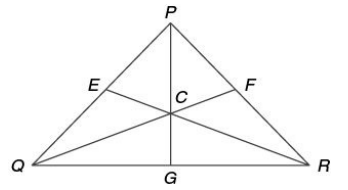
Median
A line joining the mid-point of a side of a triangle to the opposite vertex is called a median. In the figure the three medians are PG, QF and RE where G, E and F are mid-points of their respective sides.
A median divides a triangle into two parts of equal area
The point where the three medians of a triangle meet is called the centroid of the triangle.
The centroid of a triangle divides each median in the ratio 2 : 1. i.e. PC : CG = 2 : 1 = QC : CF = RC : CE
2 × (median)2 + 2 × (1/2 the third side)2 = Sum of the squares of other two sides
2(PG)2 + 2 × \( (\frac{QR}{2})^2 = (PQ)^2 + (PR)^2 \)
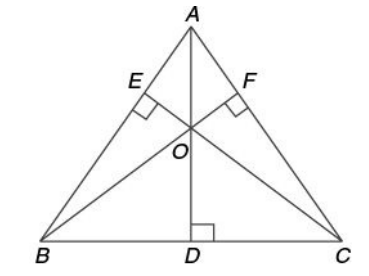
Altitude/Height
A perpendicular drawn from any vertex to the opposite side is called the altitude. (In the figure, AD, BF and CE are the altitudes of the triangles).
All the altitudes of a triangle meet at a point called the orthocentre of the triangle
The angle made by any side at the orthocentre and the vertical angle make a supplementary pair (i.e. they both add up to 180°).
∠A + ∠BOC = 180° = ∠C + ∠AOB
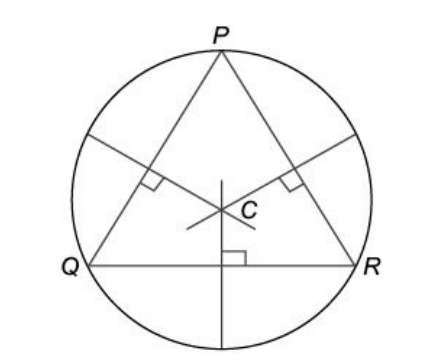
Perpendicular Bisectors
A line that is a perpendicular to a side and bisects it is the perpendicular bisector of the side.
The point at which the perpendicular bisectors of the sides meet is called the circumcentre of the triangle
The circumcentre is the centre of the circle that circumscribes the triangle. There can be only one such circle.
Angle formed by any side at the circumcentre is two times the vertical angle opposite to the side. This is the property of the circle whereby angles formed by an arc at the centre are twice that of the angle formed by the same arc in the opposite arc. Here we can view this as: ∠QCR = 2 * ∠QPR (when we consider arc QR and it’s opposite arc QPR)
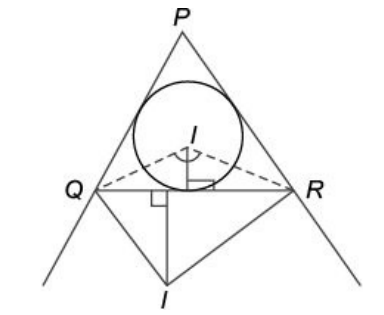
Incenter
The lines bisecting the interior angles of a triangle are the angle bisectors of that triangle. The angle bisectors meet at a point called the incentre of the triangle. The incentre is equidistant from all the sides of the triangle.
From the incentre with a perpendicular drawn to any of the sides as the radius, a circle can be drawn touching all the three sides. This is called the incircle of the triangle. The radius of the incircle is known as inradius.
The angle formed by any side at the incentre is always a right angle more than half the angle opposite to the side. This can be illustrated as ∠QIR = 90 + 1/2 ∠P
If QI and RI be the angle bisectors of exterior angles at Q and R then, QIR = 90 – 1/2 ∠P.
QUADRILATERALS
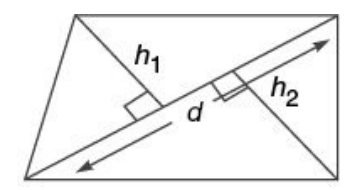
Area = 1/2 (product of diagonals) × (sine of the angle between them)
If θ1 and θ2 are the two angles made between themselves by the two diagonals, we have by the property of intersecting lines : θ1 + θ2 = 180°
Area = 1/2 × diagonal × sum of the perpendiculars to it from opposite vertices = \( \frac{d(h_1 + h_2)}{2} \)
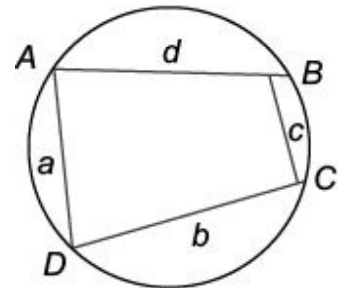
Area of a circumscribed quadrilateral = \( A = \sqrt{(S-a)(S-b)(S-c)(S-d)} \) where S = \( \frac{a+b+c+d}{2} \) (where a, b, c and d are the lengths of the sides.)

TYPES OF QUADRILATERALS - Parallelogram (|| gm)
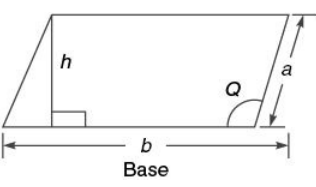
A parallelogram is a quadrilateral with opposite sides parallel (as shown in the figure below.) Area = Base (b) × Height (h)
Area = product of any two adjacent sides × sine of the included angle = ab sin Q
Perimeter = 2 (a + b) where a and b are any two adjacent sides
Diagonals of a parallelogram bisect each other.
Bisectors of the angles of a parallelogram form a rectangle.
A parallelogram inscribed in a circle is a rectangle.
A parallelogram circumscribed about a circle is a rhombus.
The opposite angles in a parallelogram are equal.
The sum of the squares of the diagonals is equal to the sum of the squares of the four sides in the figure: \( AC^2 + BD^2 = 2(AB^2 + BC^2) \).

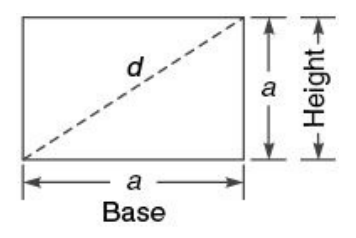
Rectangles
A rectangle is a parallelogram with all angles 90°
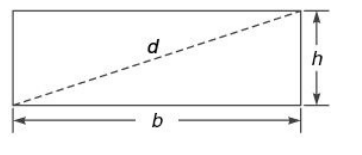
Area = Base × Height = b × h
Base and height are also referred to as the length and the breadth in a rectangle.
Diagonal (d) = \( \sqrt{b^2 + h^2} \) by Pythagoras theorem
Diagonals are equal and bisect each other
Bisectors of the angles of a rectangle (a parallelogram) form another rectangle.
All rectangles are parallelograms but the reverse is not true.
Rhombus
A parallelogram having all the sides equal is a rhombus
Area = 1/2 × product of diagonals × sine of the angle between them = \( 1/2 * d_1 * d_2\)
(Diagonals in a rhombus intersect at right angles)
Area = product of adjacent sides × sine of the angle between them.
Diagonals bisect each other at right angles.
All rhombuses are parallelograms but the reverse is not true
A rhombus may or may not be a square but all squares are rhombuses.
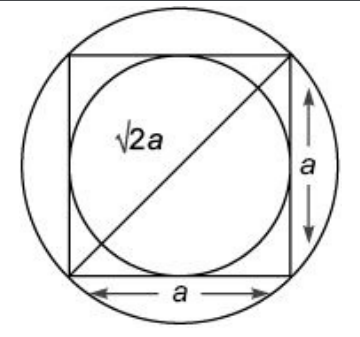
Square
A square is a rectangle with adjacent sides equal or a rhombus with each angle 90°
Area = base × height = a2
Area = 1/2 (diagonal)2 = 1/2 d2 (square is a rhombus too)
Perimeter = 4a (a = side of the square)
Diagonal = \( a * \sqrt{2}\)
Radius = \( \frac{a}{2} \)
Diagonals are equal and bisect each other at right angles
Side is the diameter of the inscribed circle
Diagonal is the diameter of the circumscribing circle.
Diameter = \( a * \sqrt{2}\); Circumradius = \( a / \sqrt{2}\)
Trapezium
A trapezium is a quadrilateral with only two sides parallel to each other.
Area = 1/2 * sum of parallel sides * height = 1/2 (AB + DC) * h
Median = 1/2 * sum of the parallel sides (median is the line equidistant from the parallel sides) For any line EF parallel to AB
EF = \( \frac{[P * (AB)] + [Q * (DC)]}{AD} \)
If the non-parallel sides are equal then diagonals will be equal too.

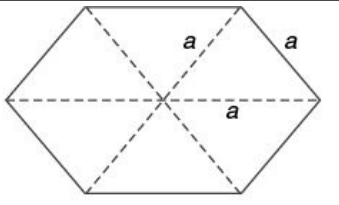
REGULAR HEXAGON
Area = \( \frac{3\sqrt{3} * a^2}{2} \)
A regular hexagon is actually a combination of 6 equilateral triangles all of side ‘a’. Hence, the area is also given by: 6 × side of equilateral triangles = 6 × \( \frac{\sqrt{3} * a^2}{4} \)
If you look at the figure closely it will not be difficult to realise that circumradius (R) = a; i.e the side of the hexagon is equal to the circumradius of the same.
CIRCLES
Area = pi * r2
Circumference = 2 pi * r = (r = radius)
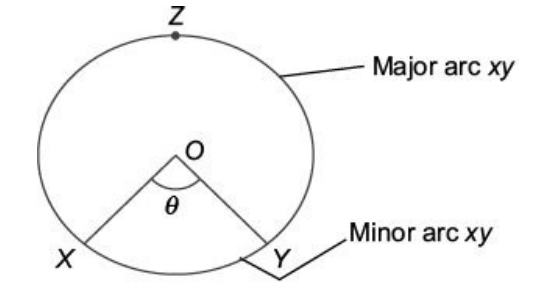
Area = 1/2 × circumference × r. Arc: It is a part of the circumference of the circle. The bigger one is called the major arc and the smaller one the minor arc.
Length (Arc XY) = \( \frac{\theta}{360} * 2*\pi*r \)
Sector of a circle is a part of the area of a circle between two radii
Area of a sector = \( \frac{\theta}{360} * \pi*r^2 \) (where θ is the angle between two radii)
= (1/2)r × length (arc xy) = \( \frac{1}{2} * r * \frac{\pi * r * \theta}{360} \)
Segment: A sector minus the triangle formed by the two radii is called the segment of the circle.
Area = Area of the sector – Area △OAB = \( \frac{\theta}{360} * \pi*r^2 - 1/2 * r^2 * sin \theta\)
Perimeter of segment = length of the arc + length of segment AB
= \( \frac{\theta}{360} * \pi*r*2 + 2*r * sin \frac{\theta}{2} = \frac{\pi * r * \theta}{180} + 2*r * sin \frac{\theta}{2} \)
Congruency: Two circles can be congruent if and only if they have equal radii
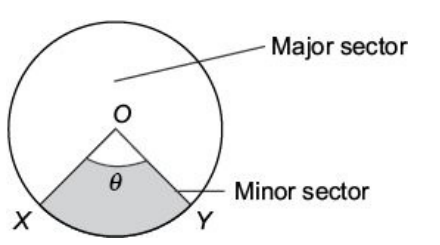
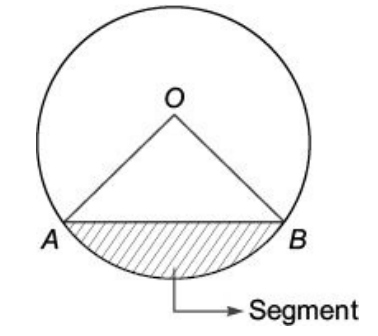
Properties of a circle
The perpendicular from the centre of a circle to a chord bisects the chord. The converse is also true.
The perpendicular bisectors of two chords of a circle intersect at its centre
There can be one and only one circle passing through three or more non-collinear points.
If two circles intersect in two points then the line through the centres is the perpendicular bisector of the common chord.
If two chords of a circle are equal, then the centre of the circle lies on the angle bisector of the two chords.
Equal chords of a circle or congruent circles are equidistant from the centre
Equidistant chords from the centre of a circle are equal to each other in terms of their length.
The degree measure of an arc of a circle is twice the angle subtended by it at any point on the alternate segment of the circle. This can be clearly seen in the following figure: With respect to the arc AB, ∠AOB = 2 ∠ACB.
Any two angles in the same segment are equal. Thus, ∠ACB = ∠ADB.
The angle subtended by a semi-circle is a right angle. Conversely, the arc of a circle subtending a right angle at any point of the circle in its alternate segment is a semi-circle.
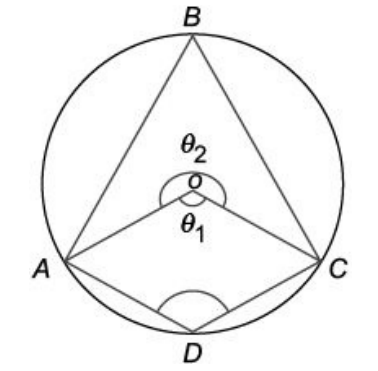
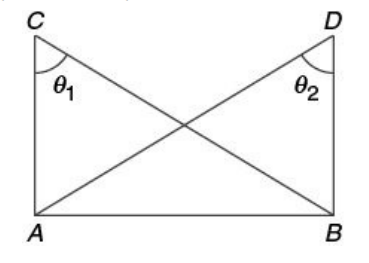
Any angle subtended by a minor arc in the alternate segment is acute, and any angle subtended by a major arc in the alternate segment is obtuse. ∠ABC is acute and ∠ADC = obtuse Also θ1 = 2 ∠B And θ2 = 2 ∠D θ1 + θ2 = 2(∠B + ∠D) = 360° = 2(∠B + ∠D) or ∠B + ∠D = 180° or sum of opposite angles of a cyclic quadrilateral is 180°
If a line segment joining two points subtends equal angles at two other points lying on the same side of the line, the four points are concyclic. Thus, in the following figure: If, θ1 = θ2 Then ABCD are concyclic, that is, they lie on the same circle.
Equal chords of a circle (or of congruent circles) subtend equal angles at the centre (at the corresponding centres.) The converse is also true
If the sum of the opposite angles of a quadrilateral is 180°, then the quadrilateral is cyclic. Secant: A line that intersects a circle at two points. Tangent: A line that touches a circle at exactly one point.
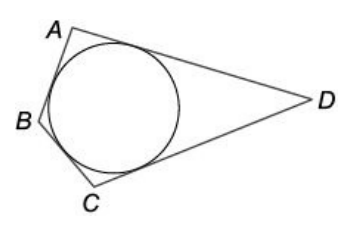
If a circle touches all the four sides of a quadrilateral then the sum of the two opposite sides is equal to the sum of other two AB + DC = AD + BC
In two concentric circles, the chord of the larger circle that is tangent to the smaller circle is bisected at the point of contact.
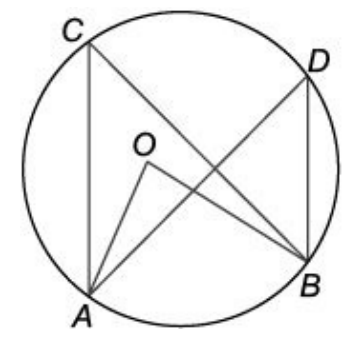
Tangents
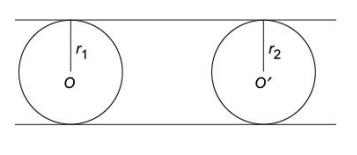
Length of direct common tangents is \( \sqrt{(\text{Distance between their centers})^2 - (r_1 - r_2)^2} \) where r1 and r2 are the radii of the circles \( \sqrt{(OO')^2 - (r_1 - r_2)^2} \)
Length of transverse common tangents is \( \sqrt{(\text{Distance between their centers})^2 - (r_1 + r_2)^2} \) where r1 and r2 are the radii of the circles \( \sqrt{(OO')^2 - (r_1 + r_2)^2} \)

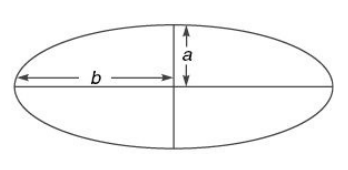
Ellipse and Star
Perimeter = p (a + b)
Area = pab
Sum of angles of a star = (2n – 8) × p/2 = (n – 4)p
PART II: MENSURATION
Cuboid
A cuboid is a three dimensional box. It is defined by the virtue of it’s length l, breadth b and height h. It can be visualised as a room which has its length, breadth and height different from each other.
Total surface area of a cuboid = 2 (lb + bh + lh)
Volume of the cuboid = lbh
Cube of side s
A cube is a cuboid which has all its edges equal i.e. length = breadth = height = s
Total surface area of a cube = 6s2.
Volume of the cube = s3.
Prism
A prism is a solid which can have any polygon at both its ends. It’s dimensions are defined by the dimensions of the polygon at it’s ends and its height.
Lateral surface area of a right prism = Perimeter of base * height
Volume of a right prism = area of base* height
Whole surface of a right prism = Lateral surface of the prism + the area of the two plane ends
Cylinder
A cylinder is a solid which has both its ends in the form of a circle. Its dimensions are defined in the form of the radius of the base (r) and the height h. A gas cylinder is a close approximation of a cylinder.
Curved surface of a right cylinder = 2prh where r is the radius of the base and h the height.
Whole surface of a right circular cylinder = 2prh + 2pr2
Volume of a right circular cylinder = pr2h
Pyramid
A pyramid is a solid which can have any polygon as its base and its edges converge to a single apex. Its dimensions are defined by the dimensions of the polygon at its base and the length of its lateral edges which lead to the apex. The Egyptian pyramids are examples of pyramids.
Slant surface of a pyramid = 1/2 * Perimeter of the base* slant height
Whole surface of a pyramid = Slant surface + area of the base
Volume of a pyramid = area of the base * 3 / heights
Cone
A cone is a solid which has a circle at its base and a slanting lateral surface that converges at the apex. Its dimensions are defined by the radius of the base (r), the height (h) and the slant height (l). A structure similar to a cone is used in ice cream cones
Curved surface of a cone = prl where l is the slant height
Whole surface of a cone = prl + pr2
Volume of a cone = \( \frac{\pi*r^2*h}{3} \)
Sphere
A sphere is a solid in the form of a ball with radius r.
Surface Area of a sphere = 4 * π * r
Volume of a sphere = 4/3 * π r3
Frustum of a pyramid
When a pyramid is cut the left over part is called the frustum of the pyramid.
Slant surface of the frustum of a pyramid = 1/2 * sum of perimeters of end * slant height.
Volume of the frustum of a pyramid = \( \frac{h}{3} [B_1 + \sqrt{(B_1*B_2)} + B_2] \) where h is the thickness and B1, B2 the areas of the ends

Frustum of a cone
When a cone is cut the left over part is called the frustum of the cone
Slant surface of the frustum of a cone = p(r1 + r2)l where l is the slant height.
Volume of the frustum of a cone = \( \frac{\pi}{3} * k * [(r_1)^2 + (r_1*r_2) + (r_2)^2] \)
Solved Question Papers
Ans .
100
- Explanation :
When the length of stick = 20 m, then length of shadow = 10 m i.e. in this case length = 2 × shadow with the same angle of inclination of the sun, the length of tower that casts a shadow of 50 m fi 2 × 50 m = 100 m i.e. height of tower = 100 m
- Explanation :
△ ABC ~ △ EDC ; then \( \frac{AC}{EC} = \frac{BC}{DC} = \frac{AB}{ED}\). Then AC/4.2 = 4/3 so AC = 5.6cm also BC/4.8 = 4/3 so BC = 6.4cm
- Explanation :
For similar triangles we have (Ratio of sides)2 = Ratio of areas Then as per question = (36/x)2 = 144/81. {Let the longest side of △ DEF = x}. So. 36/x = 12/9 so x = 27 cm
- Explanation :
(Ratio of corresponding sides)2 = Ratio of area of similar triangle \ Ratio of corresponding sides in this question. \(sqrt{\frac{16}{25} = 4/5}\)
- Explanation :
Ratio of corresponding sides = \(sqrt{\frac{9}{16}} = 3/4\)
- Explanation :
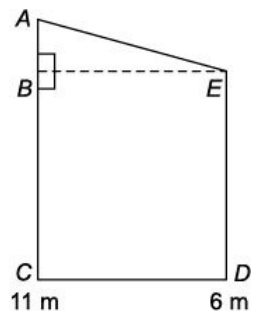
BC = ED = 6 m So AB = AC – BC = 11 – 6 = 5 m CD = BE = 12 m Then by Pythagoras theorem: AE2 = AB2 + BE2 so AE = 13 m
- Explanation :
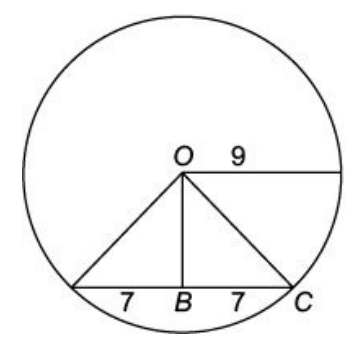
In the DOBC; BC = 7 cm and OC = 9 cm, then using Pythagoras theorem. OB2 = OC2 – BC2 OB = \( \sqrt{32} \)= 5.66 cm (approx)
- Explanation :
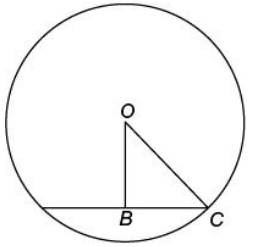
In the △ OBC, OB = 12 cm, OC = radius = 13 cm. Then using Pythagoras theorem; BC2 = OC2 – OB2 = 25; BC = 5 cm Length of the chord = 2 × BC = 2 × 5 = 10 cm
- Explanation :
x = 35°; because angles subtended by an arc, anywhere on the circumference are equal.
- Explanation :
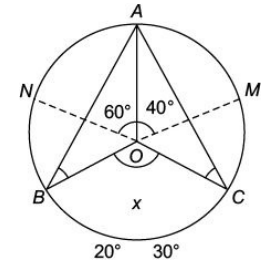 ∠AOM = 2∠ABM and
∠AON = 2∠ACN
because angle subtended by an arc at the centre of the circle is twice the angle subtended by it on
the circumference on the same segment.
∠AON = 60° and ∠AOM = 40°
∠X = ∠AON + ∠AOM
(vertically opposite angles).
∠X = 100
∠AOM = 2∠ABM and
∠AON = 2∠ACN
because angle subtended by an arc at the centre of the circle is twice the angle subtended by it on
the circumference on the same segment.
∠AON = 60° and ∠AOM = 40°
∠X = ∠AON + ∠AOM
(vertically opposite angles).
∠X = 100
- Explanation :
△BCO and △AOD are similar (AC and BD one diameters). Thus ∠ADO = ∠CBD (alternate opposite angles) ∠X = 30°
- Explanation :
By the rule of tangents, we know: 62 = (5 + n)5 so 36 = 25 + 5x so 11 = 5x so x = 2.2 cm
- Explanation :
By the rule of tangents, we get 122 = (x + 7)x so 144 = x2 + 7x fi x2 + 7x – 144 = 0 fi x2 + 16x – 9x – 144 = 0 fi x(x + 16) – 9(x + 16) fi x = 9 or –16 –16 can’t be the length, hence this value is discarded, thus, x = 9
- Explanation :
By the rule of chords, cutting externally, we get fi (9 + 6)6 = (5 + x)5 fi 90 = 25 + 5x fi 5x = 65 fi x = 13 cm
- Explanation :
Inradius = area/semi perimeter = 24/12 = 2 cm
- Explanation :
∠APB = 90° (angle in a semicircle = 90°) ∠PBA = 180 – (90 + 25) = 65° ∠TPA = ∠PBA(the angle that a chord makes with the tangent, is subtended by the chord on the circumference in the alternate segment) = 65°
- Explanation :
ADBC is a cyclic quadrilateral as all its four vertices are on the circumference of the circle. Also, the opposite angles of the cyclic quadrilateral are supplementary. Therefore, ∠ADB = 180 – 48° = 132°
- Explanation :
∠POS = ∠QOR (vertically opposite angles) So a = 4b ∠SOT + ∠TOQ + ∠QOR = 180° (sum of angles on a line = 180°) 4b + 2c = 180° 84 + 2c = 180° fi 2c = 96° fi c = 48° So a = 84°, b = 21°, c = 48°
- Explanation :
∠ABC = 180° – 130° = 50° (\ sum of angles on a line = 180°) ∠ADC = 180° – ∠ABC = 130° (opposite angles of a cyclic quadrilateral are supplementary). ∠x = 180° – 130° = 50° (\ sum of angles on a line = 180°)
- Explanation :
 ∠ADC = = 70° (because the angle subtended by an arc on the circumference is half of what it
subtends at the centre). ABCD one cyclic quadrilateral
So ∠ABC = 180° – 70° = 110° (because opposite angles of a cyclic quadrilateral are
supplementary).
∠ADC = = 70° (because the angle subtended by an arc on the circumference is half of what it
subtends at the centre). ABCD one cyclic quadrilateral
So ∠ABC = 180° – 70° = 110° (because opposite angles of a cyclic quadrilateral are
supplementary).
- Explanation :
 OB = OA = radius of the circle
∠AOB = 180 – (30 + 30)
{Sum of angles of a triangle = 180°)
fi 120°
Then ∠ADB = = 60°; because the angle subtended by a chord at the centre is twice of what it
can subtend at the circumference. Again, ABCD is a cyclic quadrilateral;
So ∠ACB = 180° – 60° = 120° (because opposite angles of cyclic quadrilateral are
supplementary).
OB = OA = radius of the circle
∠AOB = 180 – (30 + 30)
{Sum of angles of a triangle = 180°)
fi 120°
Then ∠ADB = = 60°; because the angle subtended by a chord at the centre is twice of what it
can subtend at the circumference. Again, ABCD is a cyclic quadrilateral;
So ∠ACB = 180° – 60° = 120° (because opposite angles of cyclic quadrilateral are
supplementary).
- Explanation :
∠BAC = 30° (angles subtended by an arc anywhere on the circumference in the same segment are equal). In △BAC; ∠x = 180° – (110° + 30°) = 40° (sum of angles of a triangle = 180°)
- Explanation :
As L4 and L3 are not parallel lines, so there can’t be any relation between 80° and x°. Hence the answer cannot be determined.
- Explanation :
 Perimeter of the figure = 10 + 10 + 6 + 3p
= 26 + 3p
Perimeter of the figure = 10 + 10 + 6 + 3p
= 26 + 3p
- Explanation :
(b)CN : BR = 4 : 3 So the required answer is 3 : 5 : 2 : 7 : 4 Option (b) is correct.
Quiz
Score more than 80% marks and move ahead else stay back and read again!