NCERT Class 12 Chapter-12: Introduction to 3-D Geometry
Exercise 12.1
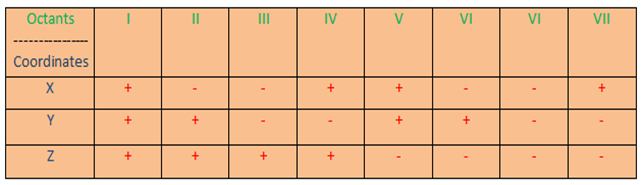
For any given point, the sign of its coordinates determines the octant in which it will lie.
Now, from the following table it can be easily determined in which coordinates the point lies.
Based on formulae given in Introduction to 3-D Geometry
Q.1: A point is lying on y – axis. What are its ‘x‘ coordinates and ‘z’ coordinates?
Sol. Based on formulae given in Introduction to 3-D Geometry
‘x’ coordinates and ‘z’ coordinates are zero if any point lies on y –axis.
Q.2: A point is lying on YZ – plane. What are its ‘x‘ coordinates?
Sol. Based on formulae given in Introduction to 3-D Geometry
If any point lies in the YZ plane then its x-coordinates are zero.
Q.3: In which of the octant the following points lie:
(2, 3, 4), (8, -1, -1), (-4, 9, -8), (-1, -2, -3), (4, -5, 6), (7, -1, -4), (-3, -5, 1), (0, 0, -3)
Sol. Based on formulae given in Introduction to 3-D Geometry
\(\Rightarrow\) The x – coordinates, y – coordinates and z – coordinates of point (2, 3, 4) are all positive. Therefore, this point is lying in octant (I).
\(\Rightarrow\) The x – coordinates, y – coordinates and z – coordinates of point (8, -1, -1) are positive, negative and negative. Therefore, this point is lying in octant (VII).
\(\Rightarrow\) The x – coordinates, y – coordinates and z – coordinates of point (-4, 9, -8) are negative, positive and negative. Therefore, this point is lying in octant (VI).
\(\Rightarrow\) The x – coordinates, y – coordinates and z – coordinates of point (-1, -2, -3) are all negative. Therefore, this point is lying in octant (VII).
\(\Rightarrow\) The x – coordinates, y – coordinates and z – coordinates of point (4, -5, 6) are positive, negative and positive. Therefore, this point is lying in octant (IV).
\(\Rightarrow\) The x – coordinates, y – coordinates and z – coordinates of point (7, -1, -4) are positive, negative and negative. Therefore, this point is lying in octant (VII).
\(\Rightarrow\) The x – coordinates, y – coordinates and z – coordinates of point (-3, -5, 1) are negative, negative and positive. Therefore, this point is lying in octant (III).
\(\Rightarrow\) The x – coordinates, y – coordinates and z – coordinates of point (-1, 2, 2) are negative, positive and positive. Therefore, this point is lying in octant (II).
Q.4: Answer the following questions:
(i). What is the name of a plane determined by the Z-axis and the Y-axis when taken together?
(ii). What is the general form of coordinates of points in the XZ-plane?
(iii). Coordinate plane divides the space into how many octants?
Sol. Based on formulae given in Introduction to 3-D Geometry
(i). The plane determined by the Z-axis and the Y-axis when taken together is known as YZ–plane.
(ii). The general form of coordinates of points in the XZ-plane is ( x, 0 , z ).
(iii). The coordinate plane divides the space into 8 octants.
Exercise: 12.2
Distance Formula:
The distance between point A(x1, y1, z1) and point B(x2, y2, z2) is given by:
AB = \(\sqrt{\left ( x_{2}-x_{1} \right )^{2}+\left ( y_{2}-y_{1} \right )^{2}+\left ( z_{2}- z_{1}\right )^{2}}\)
Q.1: Find the distance between two points whose coordinates are given below:
(i). (2, 8, 9) and (4, 5, 8)
(ii). (-3, 4, 5) and (2, 6, -1)
(iii). (-6, -4, 1) and (5, -2, 6)
(iv). (-1, 9, 8) and (6, 5, -3)
Sol. Based on formulae given in Introduction to 3-D Geometry
The distance between point A (x1, y1, z1) and point B (x2, y2, z2) is given by:
AB = \(\sqrt{\left ( x_{2}-x_{1} \right )^{2}+\left ( y_{2}-y_{1} \right )^{2}+\left ( z_{2}- z_{1}\right )^{2}}\\\)
(i). The distance between point A (2, 8, 9) and point B (4, 5, 8) is:
\(AB = \sqrt{\left ( 4-2 \right )^{2}+\left ( 5-8 \right )^{2}+\left ( 8-9\right )^{2}}\\\) \(\\\Rightarrow AB = \sqrt{4+9+1}\)\(\\\Rightarrow AB = \sqrt{14}\) units
Therefore, The distance between point A (2, 8, 9) and point B (4, 5, 8) is \(\sqrt{14}\) units
(ii). The distance between point A (-3, 4, 5) and point B (2, 6, -1) is:
\(AB = \sqrt{\left [ 2-(-3) \right ]^{2}+\left ( 6-4 \right )^{2}+\left ( -1-5\right )^{2}}\\\)\(\\\Rightarrow\) \(AB = \sqrt{25+4+36}\)
\(\\\Rightarrow\) \(AB = \sqrt{65}\) units
Therefore, The distance between point A (-3, 4, 5) and point B (2, 6, -1) is \(\sqrt{65}\) units
(iii). The distance between point A (-6, -4, 1) and point B (5, -2, 6) is:
\(AB = \sqrt{\left [ 5-(-6) \right ]^{2}+ [-2-(-4)]^{2}+\left ( 6-1\right )^{2}}\\\)\(\\\Rightarrow\) \(AB = \sqrt{121+4+25}\)
\(\\\Rightarrow\) \( AB = \sqrt{150}\) units
Therefore, The distance between point A (-6, -4, 1) and point B (5, -2, 6) is \(5\sqrt{6}\) units
(iv). The distance between point A (-1, 9, 8) and point B (6, 5, -3) is:
\(AB = \sqrt{\left [ 6-(-1) \right ]^{2}+ (5-9)^{2}+\left ( -3-8\right )^{2}}\\\)\(\\\Rightarrow\) \(AB = \sqrt{49+16+121}\)
\(\\\Rightarrow\) \(AB = \sqrt{186}\) units
Therefore, The distance between point A (-1, 9, 8) and point B (6, 5, -3) is \(\sqrt{186}\) units
Q.2: Show that the points (7, 0, -1), (-2, 3, 5) and (1, 2, 3) are collinear.
Sol. Based on formulae given in Introduction to 3-D Geometry
Let, the coordinates of points A, B and C are (7, 0, -1), (-2, 3, 5) and (1, 2, 3) respectively.
Points A, B and C are collinear if they lie on the same line.
Now, \(AB=\sqrt{(-2-7)^{2}+(3-0)^{2}+[5-(-1)]^{2}}\)
\(\Rightarrow \) \(AB=\sqrt{81+9+36}\)
\(\Rightarrow AB=\sqrt{126}\) units
Therefore, AB = \(3\sqrt{14}\) units
Now, \(BC=\sqrt{[1-(-2)]^{2}+(2-3)^{2}+(3-5)^{2}}\)
\(\Rightarrow\) \(BC=\sqrt{9+1+4}\)
\(\Rightarrow\) \(BC=\sqrt{14}\) units
Therefore, BC = \(\sqrt{14}\) units
And, \(AC=\sqrt{(1-7)^{2}+(2-0)^{2}+[3-(-1)]^{2}}\)
\(\Rightarrow\) \(AC=\sqrt{36+4+16}\)
\(\Rightarrow AC=\sqrt{56}\) units
Therefore, AC = \(2\sqrt{14}\) units
Since, AC + BC = \(2\sqrt{14}+\sqrt{14}\) = AB
Therefore, the points (7, 0, -1), (-2, 3, 5) and (1, 2, 3) are collinear.
Q.3: Prove the following statements:
(i). (-4, 9, 6), (0, 7, 10) and (-1, 6, 6) are the vertices of a right angled triangle.
(ii). (4, 9, -6), (0, 7, -10) and (1, 6, -6) are the vertices of an isosceles triangle.
(iii). (2, -3, 4), (1, -2, 5), (-1, 2, 1) and (4, -7, 8) are the vertices of a parallelogram.
Sol Based on formulae given in Introduction to 3-D Geometry
The distance between points A (x1, y1, z1) and B (x2, y2, z2) is given by:
AB = \(\sqrt{\left ( x_{2}-x_{1} \right )^{2}+\left ( y_{2}-y_{1} \right )^{2}+\left ( z_{2}- z_{1}\right )^{2}}\)
(i). Let, the coordinates of points A, B and C are (-4, 9, 6), (0, 7, 10) and (-1, 6, 6) respectively.
Now, \(AB=\sqrt{(0+4)^{2}+(7-9)^{2}+(10-6)^{2}}\\\)
\(\\\Rightarrow\) \(AB=\sqrt{16+4+16} =\sqrt{36}\) units.
Therefore, AB = 6 units.
Now, \(BC=\sqrt{(-1-0)^{2}+(6-7)^{2}+(6-10)^{2}}\\\)
\(\\\Rightarrow \) \(BC=\sqrt{1+1+16} \;=\sqrt{18}\) units.
Therefore, BC = \(3\sqrt{2}\\\) units.
And, \(AC=\sqrt{(-1+4)^{2}+(6-9)^{2}+(6-6)^{2}}\\\)
\(\\\Rightarrow\) \(AC=\sqrt{9+9}=\sqrt{18}\) units.
Therefore, AC = \(3\sqrt{2}\)units.
Now, AB2 = 36, BC2 = 18 and AC2 = 18
Since, AB2 = AC2 + BC2. Therefore, by Pythagoras theorem, ABC is a right angled triangle.
Hence, the given points (-4, 9, 6), (0, 7, 10) and (-1, 6, 6) are the vertices of a right angled triangle.
(ii). Let, the coordinates of points A, B and C are (4, 9, -6), (0, 7, -10) and (1, 6, -6) respectively.
Now, \(AB=\sqrt{(0-4)^{2}+(7-9)^{2}+(-10+6)^{2}}\\\)
\(\\\Rightarrow\) \(AB=\sqrt{36}\) units.
Therefore, AB = 6 units.
Now, \(BC=\sqrt{(1-0)^{2}+(6-7)^{2}+(-6+10)^{2}}\\\)
\(\\\Rightarrow\) \(BC=\sqrt{1+1+16} =\sqrt{18}\) units.
Therefore, BC = \(3\sqrt{2}\) units.
And, \(AC=\sqrt{(1-4)^{2}+(6-9)^{2}+(-6+6)^{2}}\\\)
\(\\\Rightarrow AC=\sqrt{9+9}=\sqrt{18}\) units.
Therefore, AC = \(3\sqrt{2}\) units.
i.e. AC = BC ≠ AB
Now, a triangle is said to be an isosceles triangle if two sides of a triangle are equal to each other.
Since, AC = BC. Therefore triangle ABC is an isosceles triangle.
Hence, the given points (4, 9, -6), (0, 7, -10) and (1, 6, -6) are the vertices of an isosceles triangle.
(iii). Let, the coordinates of points A, B, C and D are (1, -2, 5), (4, -7, 8), (2, -3, 4) and (-1, 2, 1) respectively.
Now, \(AB=\sqrt{(4-1)^{2}+(-7+2)^{2}+(8-5)^{2}}\\\)
\(\\\Rightarrow\) \(AB=\sqrt{9+25+9} =\sqrt{43}\) units.
Therefore, AB =\(\sqrt{43}\) units.
Now, \(BC=\sqrt{(2-4)^{2}+(-3+7)^{2}+(4-8)^{2}}\\\)
\(\\\Rightarrow\) \(BC=\sqrt{4+16+16} =\sqrt{36}\) units.
Therefore, BC = 6 units.
Now, \(CD =\sqrt{(-1-2)^{2}+(2+3)^{2}+(1-4)^{2}}\)
\(\\\Rightarrow \) \(AC=\sqrt{9+25+9} =\sqrt{433}\) units.
Therefore, CD = \(\sqrt{43}\) units.
And, \(AD =\sqrt{(-1-1)^{2}+(2+2)^{2}+(1-5)^{2}}\\\)
\(\\\Rightarrow\) \(AD=\sqrt{4+16+16} =\sqrt{36}\) units.
Therefore, AD = 6 units.
Since, BC = AD = 6 units and AB = CD = \(\sqrt{43}\) units
Since, it is proved that in quadrilateral ABCD opposite sides are equal. Therefore, ABCD is a parallelogram.
Hence, the given points (2, -3, 4), (1, -2, 5), (-1, 2, 1) and (4, -7, 8) are the vertices of a parallelogram.
Q.4: Find the equation of the set of points P which are equidistant from point A (3, 2, 1) and point B (-1, 2, 3).
Sol. Based on formulae given in Introduction to 3-D Geometry
Let, (x, y, z) be the coordinates of point P, which is equidistant from point A (3, 2, 1) and point B (-1, 2, 3).
Now, according to the given condition:
AP2 = BP2
\(\Rightarrow\) \(\left [ \sqrt{(x-3)^{2}+(y-2)^{2}+(z-1)^{2}} \;\right ]^{2}=\left [ \sqrt{(x+1)^{2}+(y-2)^{2}+(z-3)^{2}} \;\right ]^{2}\)
\(\\\Rightarrow\) \((x-3)^{2}+(y-2)^{2}+(z-1)^{2}=(x+1)^{2}+(y-2)^{2}+(z-3)^{2}\)
\(\\\Rightarrow\) \(x^{2}+9-6x+y^{2}+4-4y+z^{2}+1-2z=x^{2}+1+2x+y^{2}+4-4y+z^{2}+9-6z\)
\(\\\Rightarrow\) – 6x – 4y – 2z = +2x – 4y – 6z
\(\\\Rightarrow\) – 6x – 4y – 2z = + 2x – 4y – 6z
\(\\\Rightarrow\) 8x – 4z = 0
Therefore, the required equation is: 2x – z = 0
Q.5: The sum of distance of point P from point A (3, 0, 0) and point B (-3, 0, 0) is equal to 12 units. Find the equation of the set of points.
Sol. Based on formulae given in Introduction to 3-D Geometry
Let, (x, y, z) be the coordinates of point P.
Now, according to the given condition:
AP + BP = 12
\(\Rightarrow\) \(\sqrt{(x-3)^{2}+y^{2}+z^{2}}+\sqrt{[x-(-3)]^{2}+y^{2}+z^{2}}=12\\\)
\(\Rightarrow\) \(\sqrt{(x-3)^{2}+y^{2}+z^{2}}=12-\sqrt{(x+3)^{2}+y^{2}+z^{2}}\)
Now, on squaring both the side, we will get:
\(\Rightarrow\) \(\left ( \sqrt{(x-3)^{2}+y^{2}+z^{2}} \right )^{2}= \left ( 12-\sqrt{(x+3)^{2}+y^{2}+z^{2}} \right )^{2}\\\)
\(\Rightarrow\) \((x-3)^{2}+y^{2}+z^{2}= 144+[(x+3)^{2}+y^{2}+z^{2}]-24\sqrt{(x+3)^{2}+y^{2}+z^{2}}\\\)
\(\Rightarrow\) \(x^{2}+9-6x+y^{2}+z^{2}=144+x^{2}+9+6x+y^{2}+z^{2}-24\sqrt{(x+3)^{2}+y^{2}+z^{2}}\\\)
\(\Rightarrow\) \(24\sqrt{(x+3)^{2}+y^{2}+z^{2}}=144+12x\\\)
\(\Rightarrow\) \(2\sqrt{(x+3)^{2}+y^{2}+z^{2}}=(x+12)\)
Again, on squaring both the side, we will get:
\(\Rightarrow\) \(4[(x+3)^{2}+y^{2}+z^{2}]=(x+12)^{2}\\\)
\(\Rightarrow\) \(4x^{2}+4y^{2}+4z^{2}+36+24x=x^{2}+24x+144\\\)
\(\Rightarrow\) \(3x^{2}+4y^{2}+4z^{2}-108=0\)
Therefore, the required equation is: \(3x^{2}+4y^{2}+4z^{2}-108=0\)
Section Formula:
The coordinates of point P, which divides the line segment joining two points A (a1, b1, c1) and B (a2, b2, c2) internally in the ratio m and n are:
\(\left [ \frac{m.a_{2}\;+\;n.a_{1}}{m\;+\;n},\frac{m.b_{2}\;+\;n.b_{1}}{m\;+\;n},\frac{m.c_{2}\;+\;n.c_{1}}{m\;+\;n} \right ]\)
The coordinates of point Q, which divides the line segment joining two points A (a1, b1, c1) and B (a2, b2, c2) Externally in the ratio m and n are:
\(\left [ \frac{m.a_{2}\;-\;n.a_{1}}{m\;-\;n},\frac{m.b_{2}\;-\;n.b_{1}}{m\;-\;n},\frac{m.c_{2}\;-\;n.c_{1}}{m\;-\;n} \right]\)
Exercise 12.3
Q.1: Find the coordinates of the point which divides the line segment joining the points (-2, 1, 0) and (1, 3, 6) internally in the ratio of 1:4 and externally in the ratio of 1:4.
Sol: Based on formulae given in Introduction to 3-D Geometry
Since, the coordinates of point P, which divides the line segment joining two points A (a1, b1, c1) and B (a2, b2, c2) internally in the ratio m and n are:
\(\left [ \frac{m.a_{2}\;+\;n.a_{1}}{m\;+\;n},\frac{m.b_{2}\;+\;n.b_{1}}{m\;+\;n},\frac{m.c_{2}\;+\;n.c_{1}}{m\;+\;n} \right ]\)
Therefore, the coordinates of point P, which divides the line segment joining two points A(-2, 1, 0) and B (1, 3, 6) internally in the ratio 1 and 4 are:
(x, y, z) = \(\left [ \frac{1\times 1\;+\;4\times -2}{1\;+\;4},\frac{1\times 3\;+\;4\times 1}{1\;+\;4},\frac{1\times 6\;+\;4\times 0}{1\;+\;4} \right ]\\\)
(x, y, z) = \(\left [ \frac{-7}{5},\frac{7}{5},\frac{6}{5} \right ]\)
Therefore, the coordinates of point P are: \(\left [ \frac{-7}{5},\frac{7}{5},\frac{6}{5} \right ]\\\)
Now, The coordinates of point Q, which divides the line segment joining two points A (a1, b1, c1) and B (a2, b2, c2) Externally in the ratio m and n are:
\(\left [ \frac{m.a_{2}\;-\;n.a_{1}}{m\;-\;n},\frac{m.b_{2}\;-\;n.b_{1}}{m\;-\;n},\frac{m.c_{2}\;-\;n.c_{1}}{m\;-\;n} \right]\)
Therefore, the coordinates of point Q, which divides the line segment joining two points A(-2, 1, 0) and B (1, 3, 6) externally in the ratio 1 and 4 are:
(x, y, z) = \(\left [ \frac{1\times 1\;-\;4\times -2}{1\;-\;4},\frac{1\times 3\;-\;4\times 1}{1\;-\;4},\frac{1\times 6\;-\;4\times 0}{1\;-\;4} \right ]\\\)
(x, y, z) = \(\left [ \frac{9}{-3},\frac{-1}{-3},\frac{6}{-3} \right ]\)
Therefore, the coordinates of point Q are: \(\left [ -3,\frac{1}{3},-2 \right ]\)
Q.2: Given that the points A (3, 2, -4) B (5, 4, -6) and C (9, 8, -10), are collinear. Find the ratio in which line AC is divided by B.
Sol. Based on formulae given in Introduction to 3-D Geometry
Since, point A, point B and point C are collinear. Therefore, let us assume that point B divides the line segment joining point A and point C in the ratio k : 1.
Hence, by Section Formula, coordinates of point B are:
\(\Rightarrow \left [ \frac{(k\times 9)\;+\;(1\times 3)}{k\;+\;1},\frac{(k\times 8)\;+\;(1\times 2)}{k+1},\frac{(k\times -10)\;+\;(1\times -4)}{k\;+\;1} \right ]\\\)\(\Rightarrow \left [ \frac{9k\;+\;3}{k\;+\;1},\frac{8k\;+\;3}{k\;+\;1},\frac{-10k\;-\;4}{k\;+\;1} \right ]\). . . . . . . . . . . . . . (1)
And, the coordinates of point B are: (5, 4, -6) [Given]
Therefore, on comparing it with equation (1) we will get:
\(\Rightarrow \frac{9k\;+\;3}{k\;+\;1}=5\\\)\(\Rightarrow\) 9k + 3 = 5k + 5
Therefore, k = \(\frac{1}{2}\)
Hence, point B divides AB in the ratio of 1 : 2
Q.3: Find the ratio in which XZ – plane divides the line segment AB formed by joining the points (3, -5, 8) and (-2, 4, 7).
Sol. Based on formulae given in Introduction to 3-D Geometry
Let the XZ plane divides the line segment PQ in the ratio k : 1
Hence, by Section Formula the coordinates of point of intersection are:
\(\Rightarrow \left [ \frac{(k\times -2)\;+\;(1\times 3)}{k\;+\;1},\frac{(k\times 4)\;+\;(1\times -5)}{k\;+\;1},\frac{(k\times 7)\;+\;(1\times 8)}{k\;+\;1} \right ]\\\)\(\\\Rightarrow\) \(\left [ \frac{-2k\;+\;3}{k\;+\;1},\frac{4k\;-\;5}{k\;+\;1},\frac{7k\;+\;8}{k\;+\;1} \right ]\)
Now, in XZ – plane, the y – coordinate of any point is zero.
\(\Rightarrow \frac{4k\;-\;5}{k\;+\;1}=0\\\)Therefore, \(k = \frac{5}{4}\)
Hence, the XZ plane divides the line segment formed by the joining of given points in the ratio of 2 : 3.
Q.4: By using the section formula; Show that the points P (-1, 2, 1), Q (0, \(\frac{1}{3}\), 2) and R (2, -3, 4) are collinear.
Sol. Based on formulae given in Introduction to 3-D Geometry
Let, point A divides line PQ in the ratio k : 1
Hence, by Section Formula the coordinates of point A are:
\(\Rightarrow \left [ \frac{(k\times 0)\;+\;(1\times -1)}{k\;+\;1},\frac{(k\times \frac{1}{3})\;+\;(1\times 2)}{k\;+\;1},\frac{(k\times 2)\;+\;(1\times 1)}{k\;+\;1} \right ]\\\) \(\Rightarrow \left [ \frac{-1}{k\;+\;1},\frac{\frac{k}{3}\;+\;2}{k\;+\;1},\frac{2k\;+\;1}{k\;+\;1} \right ]\\\)Therefore, the coordinates of point A are: \(\left [ \frac{-1}{k\;+\;1},\frac{k\;+\;6}{3k\;+\;3},\frac{2k\;+\;1}{k\;+\;1} \right ]\)
Now, the value of ‘k’ for which point A coincides with point R:
\(\Rightarrow \frac{-1}{k+1}= 2\\\)
\(\Rightarrow -1= 2k+2\\\)Therefore, k = \(\frac{-3}{2}\\\)
Now, for k = \(\frac{-3}{2}\), the coordinates of point A are:
\(\\\Rightarrow\) \(\left [ \frac{-1}{\left ( \frac{-3}{2} \right )\;+\;1}\;,\;\frac{\left ( \frac{-3}{2} \right )\;+\;6}{3\times \left ( \frac{-3}{2} \right )\;+\;3}\;,\;\frac{2\times \left ( \frac{-3}{2} \right )\;+\;1}{\left ( \frac{-3}{2} \right )\;+\;1} \right ]\\\)
\(\\\Rightarrow\) \(\left [ \frac{-1\times 2}{-3\;+\;2}\;,\;\frac{-3\;+\;12}{-9\;+\;6}\;,\;\frac{-6\;+\;2}{-3\;+\;2} \right ]\\\)
Therefore, the coordinates of point A are: (2, -3, 4)
Hence, the coordinates of point A coincides with the coordinates of point R.
\(\Rightarrow\)R (2, -3, 4) is a point that divides PQ externally and is same as point A.
Hence, the points P, Q and R are colinear.
Q.5: The line segment joining points A (5, 3, -6) and B (9, 15, 7) is trisected by the points P and Q, Find the coordinates of points P and Q.
Sol. Based on formulae given in Introduction to 3-D Geometry
Point P divides the line segment AB in the ratio 1 : 2 and point Q divides the line segment AB in the ratio 2 : 1.
Therefore, by section formula the coordinates of point P and point Q are:
For Point P:
\(\Rightarrow \left [ \frac{(1\times 9)\;+\;(2\times 5)}{1\;+\;2},\frac{(1\times 15)\;+\;(2\times 3)}{1\;+\;2},\frac{(1\times 7)\;+\;(2\times -6)}{1\;+\;2} \right ]\\\) \(\Rightarrow \left [ \frac{19}{3},\frac{21}{3},\frac{-5}{3} \right ]\\\)Therefore, the coordinates of point P are:\(\left [ \frac{19}{3},7,\frac{-5}{3} \right ]\)
For Point Q:
\(\Rightarrow \left [ \frac{(2\times 9)\;+\;(1\times 5)}{2\;+\;1},\frac{(2\times 15)\;+\;(1\times 3)}{2\;+\;1},\frac{(2\times 7)\;+\;(1\times -6)}{2\;+\;1} \right ]\\\) \(\Rightarrow \left [ \frac{23}{3},\frac{33}{3},\frac{8}{3} \right ]\\\)Therefore, the coordinates of point Q are: \(\left [ \frac{23}{3},11,\frac{8}{3} \right ]\)