-
Chapter 11: SERIES COMPLETION
Introduction
This involves recognizing the next term in a series and choosing one from the alternatives given.
Q. 7 , 12 , 19 , X , 39
A. X = 28 as the series follows the pattern: 7, 7+5, 12+7, 19+9, 28+11 where the number is added by the next odd number.
Q. 0, 6, 24, 60, 120, 210, X
A. X = 336 as series follows pattern 13-1 , 23-2 , 33-3 , 43-4....
Q. 4 , 6 , 12 , 14 , 28 , 30 , X
A. There are 2 series in this series 4, 12, 28 ... and 6, 14, 30 and both follow pattern +8, +16, +32, So we get missing value as 28+32 = 60
Q. 1, 4, 9, 16, 25, X
A. Series follows pattern: +3, +5, +7, +9 ...
Q. 20, 19, 17, X, 10, 5
A. Series follows pattern: -1, -2, -3, -4, -5
Q. 6, 11, 21, 36, 56...
A. Pattern: +5, +10, +15, +20, +25...
Q. 1, 6, 13, 22, 33, ...
A. Pattern: +5, +7, +9, +11 ...
Q. 1, 9, 17, 33, 49, 73, X
A. Pattern: + 4 * 2, + 4 * 2, + 4 * 4, + 4 * 4, + 4 * 6, + 4 * 6
Q. 0.5, 1.5, 4.5, 13.5, X
A. Pattern: + 1 , + 1 * 3, + 3 * 3, + 9 * 3 ; Multiply the previous value obtained by 3 each time.
Q. 19, 2, 38, 3, 114, 4, X
A. There are two series: 2, 3, 4, ... and 19, 38, 114, X. The second series follows patterns: * 2, * 3 , * 4, ... So answer is 114*4 = 456
Choose the wrong alternative
A. Series follows pattern: 23 - 1, 33-1, 43-1, 53-1 ... Now we have to check which term doesn't fit this pattern.
Q. 3, 8, 15, 24, 34, 48, 63 ...
A. Series pattern: next term - previous term = 5, 7, 9 ...
Q. 196, 169, 144, 121, 80
A. Pattern: 142, 132, 122, 112 ...
Q. 121, 143, 165, 186, 209
A. Pattern: 112 -02, 122-12, 132-22, 142 - 32, 152 - 42 . Thus odd term is 186.
Q. 1, 2, 4, 8, 16, 32, 64, 96
A. 96 as it is not double of previous
Q. 1, 3, 7, 15, 27, 63, 127
A. Series pattern: +2, +4, +8, +16 .... 27 doesn't follow this pattern.
Q. 6, 14, 30, 64, 126 ...
A. Pattern: +8, +16, +32, +64 and 64 breaks the pattern.
Q. 1236, 2346, 3456, 4566, 5686
A. 5686 breaks the pattern; The first three digits form continuous series so last number should be 5676.
Q. 5, 10, 40, 80, 320, 550, 2560
A. Series follows the pattern: * 2, * 4, * 2, * 4 ....
Q. 11, 2, 21, 3, 32, 4, 41, 5, 51, 6
A. There are two series: 2, 3, 4, 5.. 11 , 21 , 31 , 41 , 51. 32 breaks the second series.
Alphabet Series
Alphabet series sums can be solved using this diagram as reference.
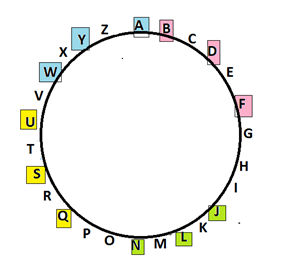
Q. ' ' , ' ', R, T, V, X, Z
A. Series starts from N and skips one alphabet.
Q. P, K, G, D, B, A
A. Series has the pattern: - 5, -4, -3 , -2 ...
Q. BDF, CFI, DHL, ....
A. The first term is moved one place ahead, second two places and third three places.
Q. YEB, WFD, UHG, SKI .....
A. The first term is moved back by 1 then 2 then 3 steps. The second and third terms are moved forward by 1 then 2 then 3 steps. Missing term :QOL
Q. AB, DG, HM, MT, SB, ...
A. The first term is moved ahead in pattern +2, +3, +4 ... The second term is moved ahead in pattern: +4, +5, +6...
Q. P3, R5, T8, V12, ...
A. The first term is moved ahead by +1, +2, +3 and second term by +2, +3, +4 ...