NCERT Chapter-16: Probability
Practice Exercise 16.1
Question 1
Write the sample space when a coin is tossed thrice.
Sol: Based on formulae given in Probability
We all know that a unbiased coin a head (H) & a tail (T)
According to the question, when a coin tossed thrice, then possible outcomes = \(2^{3}=8\)
Sample space = { TTT, HHH, HHT, THH, THT, HTH, HTT, TTH, }
Question 2
Write the sample space when a dice is rolled twice.
Sol: Based on formulae given in Probability
Possible outcomes when a die is rolled: 3, 6,4,5 &1, 2
If the die is thrown twice, the sample space = {(x,y):x,y = 1,2,3,4,5,6}
Total number of elements in the sample space = \(6^{2}=36\)
Sample space = { (1,1) , (1,2) , (1,3) , (1,4) , (1,5) , (1,6) , (5,1) , (5,2) ,( 5,3) , (5,4) , (5,5), (5,6) , (3,1) , (3,2) , (3,3) , (3,4) , (3,5) , (3,6) , (4,1) , (4,2) , (4,3) , (4,4) , (4,5) ,(4,6) , (2,1) , (2,2) , (2,3) , (2,4) ,(2,5) , (2,6) , (6,1) , (6,2) , (6,3) , (6,4) , (6,5) , (6,6) }
Question 3
Write the sample space when a coin is tossed four times.
Sol: Based on formulae given in Probability
An unbiased coin has a head (H) & a tail (T)
Accordingly, a coin is tossed 4 times, possible outcomes are = \(2^{4}=16\)
Sample space = {TTTT, TTTH ,TTHT ,TTHH , THTT ,THTH ,THHT ,THHH ,HTTT ,HTTH , HTHT , HTHH , HHTT ,HHTH , HHHT ,HHHH }
Question 4
Find the sample space of a coin and a die are tossed together.
Sol: Based on formulae given in Probability
An unbiased coin has a head (H) & a tail (T)
Possible outcomes when a die is thrown: 1, 2,3,4,5 and 6
Thus, sample space of a coin and a die when thrown together
S = {T6 , T5 , T4 , T3 ,T2 , T1 , H6 , H5 , H4 ,H3 ,H2 ,H1 }
Question 5
Find the sample space when a die is rolled and a coin is tossed only if in case there is head in the coin.
Sol: Based on formulae given in Probability
An unbiased coin has a head (H) and a tail (T)
Possible outcomes when a die is thrown: 1, 2,3,4,5 and 6
Thus, sample space of a coin and a die when thrown together
S = { T , H6 , H5 , H4 ,H3 ,H2 ,H1 }
Question 6
Room x is having 2 girls and 2 boys while Room y is having 3 girls and 1 boy. Determine the sample space where a person gets a room.
Sol: Based on formulae given in Probability
Consider 2 girls and 2 boys in room no. x be G1,G2 & B1,B2 .
Also, 3 girls and 1 boy in room no. y be G3,G4 & G5,B3 .
Sample space of the above mentioned problem is = { XG1,XG2, XG3,XG4,XG5, XB1,XB2., XB3}
Question 7
A bag contains dies of several colors such as red, white and blue. Dies are selected as random and rolled and the colour and the number in it’s uppermost face is noted down. Determine the sample space.
Sol: Based on formulae given in Probability
Possible outcomes when a die is thrown: 1, 2,3,4,5 and 6.
Let us consider red, white and blue colored dices be R , W and B respectively.
According to the question, sample space obtained when a die is rolled = { R6 , R5 , R4 , R3 , R2 , R1 , W6 , W5 , W4 , W3 , W2 , W1 , B6 , B5 , B4 , B3 , B2 , B1 }
Question 8
An experimental setup consists of a family consisting a boy and a girl with 2 children.
(i) Determine the sample space if the person conducting the experiment is interested in knowing whether it is a boy or a girl according to the order of their birth.
(ii) Determine the sample space if the person conducting the experiment is interested in knowing the number of girls in the family?
Sol: Based on formulae given in Probability
(i) Sample space depending the order of birth of a boy or a girl = { BB , BG , GB , GG }
(ii) It is said that a family can have only two children . it can either be a boy and a girl , both of them are girls or both of them are boys.
Sample space = { 2,1,0}
Question 9
A experimental setup consists of a box containing 1 red ball and 3 identical white balls. At a time 2 balls are drawn at random in succession without replacement. Find the sample space of the experimental setup.
Sol: Based on formulae given in Probability
It is given that the experimental setup contains a box containing a red ball and three identical white balls.
Sample space of the event of drawing 2 balls at random in succession without replacement is = {WW, WR, RW}
Question 10
An experimental setup consists of tossing up of a coin in the air and if head comes up it is tossed again. If a tail occurs in the first event then a die is rolled only once. Find the sample space of the experiment.
Sol: Based on formulae given in Probability
An unbiased coin has a head (H) & a tail (T)
Possible outcomes when a die is thrown: 1, 2,3,4,5 and 6
Sample space of the experimental setup = { T6 , T5 , T4 , T3 , T2 , T1 , HT , HH }
Question 11
3 bulbs are selected at a random from a bag of bulbs. Depending upon several tests each bulb is termed as defective or non-defective . Determine the sample space of this series of events.
Sol: Based on formulae given in Probability
It is given that 3 bulbs are selected at a random from a bag of bulbs. Depending upon the series of tests each bulb is termed as either non defective or defective.
Sample space of the series of events = { NNN ,NND ,NDN ,NDD , DNN , DND , DDN , DDD}
Question 12
An experiment consists of tossing a coin. During the conduction of the experiment if head comes up then a die is thrown instantly , if there is any even number on the die then it is thrown again . determine the sample space of the experiment.
Sol: Based on formulae given in Probability
An unbiased coin has a head (H) & a tail (T)
Possible outcomes when a die is thrown: 1, 2,3,4,5 and 6
Sample space of the experiment = { H66 , H65 , H64 , H63 , H62 , H61 , H46 , H45 , H44 , H42 , H41 , H26 , H25 , H24 , H23 , H22 H21 , H5 , H3 , H1 , T }
Question 13
A box containing 4 slips of paper written 1,2,3 and 4 separately. They are then mixed thoroughly and drawn one at a time twice without replacement. Determine the sample space of the experiment
Sol: Based on formulae given in Probability
If 3 appears on the first slip of paper , then the possibilities that the numbers appearing in the next slip of paper will be 1,2 and 4. If 4 appears on the first slip of paper , then the possibilities that the numbers appearing in the next slip of paper will be 1,2 and 3.
Sample space of the experiment = { (4,1) , (4,2) , (4,3) , (3,1) , (3,2) , (3,4) , (2,1) , (2,3) , (2,4) , (1,2) , (1,3) , (1,4) }
Question 14
An experiment consists of rolling a die then tossing a coin if any even number comes up while rolling the die. Also it was noted that if any odd number comes up the coin is tossed twice. Determine the sample space of the experiment.
Sol: Based on formulae given in Probability
An unbiased coin has a head (H) & a tail (T)
Possible outcomes when a die is thrown: 1, 2,3,4,5 and 6
sample space of the conducted experiment = { 5TT , 5TH , 5HT , 5HH ,3TT , 3TH , 3HT , 3HH , 1TT , 1TH ,1HT , 1HH , 6T , 6H , 4T , 4H , 2T , 2H }
Question 15
A coin is tossed if tail comes up a ball is drawn from a box containing 3 black balls and 2 red balls . A die is thrown is head comes up. Determine the sample space of the experiment.
Sol: Based on formulae given in Probability
It is given that a box contains 2 red and 3 black balls and they are named as R1,R2 and B1,B2,B3
Sample space of the experiment = { H6 , H5 , H4 , H3 , H1 , TB3 , TB2 ,TB1 , TR2 , TR 1}
Question 16
A die is rolled continuously unless and until a six shows up. Determine the sample space for this experiment.
Sol: Based on formulae given in Probability
Possible outcomes when a die is thrown: 1, 2,3,4,5 and 6
In this experiment 6 might come up in the 1st throw, 2nd throw, 3rd throw and so on …….
Therefore , the sample space of the experiment = { 6 , (1,6) , (2,6) , (3,6) , (4,6) , (5,6) , ( 1,1,6) , (1,2,6) ……… (1,5,6) , (2,1,6) , (2,2,6) ……………. (2,5,6) ………(5,1,6) , (5,2,6) }
Exercise 16.2
Question 1
An experiment consists of rolling of a die .Event E denotes that the die shows 4 and event F denotes that the die shows even number.
Are these both events that is A and B mutually exclusive?
Sol: Based on formulae given in Probability
In an experiment when a die is rolled the sample space is expressed as = {6 , 5 , 4 , 3 , 2 , 1}
According to the question,
A= {4} and B= {6, 4, 2}
It is observed from the above mentioned sample space of E and F is
= \(A\cap B\) = {4} = \(\phi\)
It is hereby observed that events A and B are not mutually exclusive events.
Question 2
An experiment consists of a die thrown in which the following events occurred:
(a) P : numbers less than 7
(b) Q : numbers larger than 7
(c) R : numbers which are product of 3
(d) S : numbers which are smaller than 4
(e) T : even numbers which are larger than 4
(f) U : numbers not less than 3
Also, \(P\cup Q\) , \(P\cap Q\) , \(Q\cup R\) , \(T\cap U\) ,
\(S\cap T\) , P – R , S – T ,
Sol: Based on formulae given in Probability
(a) P = {6, 5, 4, 3, 2, 1}
(b) Q = \(\phi\)
(c) R = {6, 3}
(d) S = {3, 2, 1}
(e) T = {6}
(f) U = {6, 5, 4, 3}
Question 3
An experiment consists throwing of a pair of dice and noting down the numbers that came up. Determine the following events:
(i) The sum of numbers is larger than 8
(ii) 2 occur on both of the die.
(iii) The sum of numbers is at least 7 and a multiple of 3.
Determine the events which are mutually exclusive?
Sol: Based on formulae given in Probability
Sample space when a pair of dice is rolled (S) = { (x,y) : x,y = 6,5,4,32,1 }
= { (6,1) , (6,2) , (6,3) , (6,4) , (6,5) , (6,6)
(5,1) , (5,2) , (5,3) , (5,4) , (5,5) , (5,6)
(4,1) , (4,2) , (4,3) , (4,4) , (4,5) , (4,6)
(3,1) , (3,2) , (3,3) , (3,4) , (3,5) , (3,6)
(2,1) , (2,2) , (2,3) , (2,4) , (2,5) , (2,6)
(1,1) , (1,2) , (1,3) , (1,4) , (1,5) , (1,6)}
P = {(4,6) , (5,4) , (3,6) , (6,4) , (6,5) (4,5) , (5,5) , (5,6) , (6,3) , (6,6) }
Q = {(6,2) , (5,2) , (4,2) , (3,2) ,(1,2) , (2,6) , (2,5) , (2,4) , (2,3) , (2,2) , (2,1) }
R = {(6,6) , (4,5) , (5,4) , (6,3) , (6,6)}
It is noted that:
\(P\cap Q\) = \(\phi\)
\(Q\cap R\) = \(\phi\)
\(R\cap P\) = {(6,6) , (6,3) ,(5,4) , (4,5) , (4,5) , (3,6) } = \(\phi\)
Therefore, events P, Q and R are mutually exclusive.
Question 4
In an experiment 3 coins at a time .Let P be the event in which there 3 heads. Q be the event in which there is 2 heads and 1 tail and R be the event in which there is 3 tails and S denotes the event in which head shows in the first coin. Find the events:
(a) Mutually exclusive?
(b) Simple?
(c) Compound?
Sol: Based on formulae given in Probability
Sample space of the experiment when 3 coins are tossed together.
S = {TTT, TTH, THT, THH, HTT, HTH, HHT, HHH}
P = {HHH}
Q = {HHT, HTH, THH}
R = {TTT}
S = {HHH, HHT, HTH, HTT}
It is noted that:
\(P\cap Q\) = \(\phi\)
\(P\cap R\) = \(\phi\)
\(P\cap S\) = {HHH} = \(\phi\)
\(Q\cap R\) = \(\phi\)
\(Q\cap S\) = {HHT , HTH} = \(\phi\)
\(R\cap S\) = \(\phi\)
(a) Events P and Q, events P and R, events Q and R and R and S are all mutually exclusive events.
(b) If an event of an experiment is having a sample point in a sample space it is known as simple event. Therefore P and Q are simple events.
(c) If an event of an experiment is having more than one sample point in a sample space it is known as compound event. Therefore Q and S are compound events.
Question 5
In an experiment 3 coins are tossed.
(a) 2 events which are mutually exclusive.
(b) 3 events which are mutually exhaustive and exclusive.
(c) 2 events, which are not mutually exclusive.
(d) 2 events which are mutually exclusive but not exhaustive.
(e) 3 events which are mutually exclusive but not exhaustive.
Sol: Based on formulae given in Probability
Sample space when 3 coins are tossed all together = {TTT, TTH, THT, THH, HTT, HTH, HHT, HHH}
(a) 2 events are said to be mutually exclusive:
P = no heads
Q = no tails
P = {TTT}, Q = {HHH} are disjoint
(b) 3 events are said to be mutually exclusive & exhaustive if :
P = no heads
Q = exactly 1 head
R = at least 2 heads
P = {TTT}
Q = {TTH, THT, HTT}
R = {THH, HTH, HHT, HHH}
Because \(P\cap Q\) = \(Q\cap R\) = \(R\cap S\) = \(\phi\) = \(P\cap Q\cap R = S\)
(c) 2 events are not mutually exclusive:
P = 3 heads
Q = at least 2 heads
P = {HHH}
Q = {THH , HTH , HHT , HHH }
Because \(P\cap Q\) = {HHH} ≠ \(\phi\)
(d) Event which are not exhaustive but are mutually exclusive:
P = exactly 1 head
Q = exactly 1 tail
P = { TTH , THT , HTT }
Q = {THH , HTH ,HHT }
Because \(P\cap Q\) = \(\phi\)
But, \(P\cup Q\) ≠ S
(e) 3 events that are not exhaustive but they are mutually exclusive:
P = exactly 3 heads
Q = 1 head and 2 tails
R = 1 tail and 2 heads
P = { HHH }
Q = { TTH , THT , HTT }
R = { THH , HTH , HHT }
Because \(P\cap Q\) = \(Q\cap R\) = \(R\cap P\) = \(\phi\)
But, \(P\cap Q\cap R ≠ S\)
Question 6
An experiment consists of throwing up of a dice and includes events A,B and C:
A = an even number on throwing of the first die
B = an odd number on throwing of the first die
C = sum of numbers on the dice will less than equals to 5.
(a) A (b) not B (c) A or B (d) A and B
(e) A but not C (f) B or C (g) B or C (h) \(A\cap B’\cap C’\)
Sol: Based on formulae given in Probability
Sample space when a pair of dice is rolled (S) = { (x,y) : x,y = 6,5,4,32,1 }
S = { (6,1) , (6,2) , (6,3) , (6,4) , (6,5) , (6,6)
(5,1) , (5,2) , (5,3) , (5,4) , (5,5) , (5,6)
(4,1) , (4,2) , (4,3) , (4,4) , (4,5) , (4,6)
(3,1) , (3,2) , (3,3) , (3,4) , (3,5) , (3,6)
(2,1) , (2,2) , (2,3) , (2,4) , (2,5) , (2,6)
(1,1) , (1,2) , (1,3) , (1,4) , (1,5) , (1,6)}
A = { (6,1) , (6,2) , (6,3) , (6,4) , (6,5) , (6,6) , (4,1) , (4,2) , (4,3) , (4,4) , (4,5) , (4,6), (2,1), (2,2) , (2,3) , (2,4) , (2,5) , (2,6) }
B = { (5,1) , (5,2) , (5,3) , (5,4) , (5,5) , (5,6) , (3,1) , (3,2) , (3,3) , (3,4) , (3,5) , (3,6), (1,1) ,(1,2) , (1,3) , (1,4) , (1,5) , (1,6) }
C = { (1,1) , (1,2) , (1,3) , (1,4), (2,1) , (2,2) , (2,3),(3,1) , (3,2) , (4,1)}
(a) A’ = {(5,1) , (5,2) , (5,3) , (5,4) , (5,5) , (5,6), (3,1) , (3,2) , (3,3) , (3,4) , (3,5) , (3,6), (1,1), (1,2) , (1,3) , (1,4) , (1,5) , (1,6) } = B
(b) Not B = B’ = { (6,1) , (6,2) , (6,3) , (6,4) , (6,5) , (6,6) , (4,1) , (4,2) , (4,3) , (4,4) , (4,5) , (4,6) , (2,1) , (2,2) , (2,3) , (2,4) , (2,5) , (2,6) } = A
(c) A or B = \(A\cup B\) = { (6,1) , (6,2) , (6,3) , (6,4) , (6,5) , (6,6)
(5,1) , (5,2) , (5,3) , (5,4) , (5,5) , (5,6)
(4,1) , (4,2) , (4,3) , (4,4) , (4,5) , (4,6)
(3,1) , (3,2) , (3,3) , (3,4) , (3,5) , (3,6)
(2,1) , (2,2) , (2,3) , (2,4) , (2,5) , (2,6)
(1,1) , (1,2) , (1,3) , (1,4) , (1,5) , (1,6)}
(d) A and B = \(A\cap B\) = \(\phi\)
(e) A but not C = A – C = {(6,1) , (6,2) , (6,3) , (6,4) , (6,5) , (6,6) , (4,2) , (4,3) , (4,4) , (4,5) , (4,6) , (2,4) , (2,5) , (2,6) }
(f) B or C = \(B\cup C\) = {(5,1) , (5,2) , (5,3) , (5,4) , (5,5) , (5,6) , (4,1) , (3,1) , (3,2) , (3,3) , (3,4) , (3,5) , (3,6) , (2,1) , (2,2) , (2,3), (1,1) , (1,2) , (1,3) , (1,4) , (1,5) , (1,6) }
(g) B and C = \(A\cap B\) = { (1,1) , (1,2) , (1,3) , (1,4) , (3,1) , (3,2) }
(h) C’ = {(6,1) , (6,2) , (6,3) , (6,4) , (6,5) , (6,6), (5,1) , (5,2) , (5,3) , (5,4) , (5,5) , (5,6) , (4,2) , (4,3) , (4,4) , (4,5) , (4,6) , (3,3) , (3,4) , (3,5) , (3,6) , (2,5) , (2,6) , (1,5) , (1,6) }
Therefore, \(A\cap B’\cap C’\) = \(A\cap A\cap C’\) = \(A\cap C’\)
= {(6,1) , (6,2) , (6,3) , (6,4) , (6,5) , (6,6) , (4,2) , (4,3) , (4,4) , (4,5) , (4,6) , (2,4) , (2,5) , (2,6)}
Question 7
An experiment consists of throwing up of a dice and includes A, B and C.
A = an even number on throwing of the first die
B = an odd number on throwing of the first die
C = sum of numbers on the dice will less than equals to 5.
Give answer in true or false.
(a) B and A are mutually exclusive events.
(b) B and A are mutually exclusive and exhaustive events.
(c) A = B’
(d) A and C are mutually exclusive and exhaustive events.
(e) B’ and A are mutually exclusive events.
(f) A’ , B’ & C are mutually exhaustive and exclusive.
Sol: Based on formulae given in Probability
A = {(6,1) , (6,2) , (6,3) , (6,4) , (6,5) , (6,6) , (2,1) , (2,2) , (2,3) , (2,4) , (2,5) , (2,6) , (4,1) , (4,2) , (4,3) , (4,4) , (4,5) , (4,6)}
B= {(5,1) , (5,2) , (5,3) , (5,4) , (5,5) , (5,6) , (3,1) , (3,2) , (3,3) , (3,4) , (3,5) , (3,6) , (1,1) , (1,2) , (1,3) , (1,4) , (1,5) , (1,6)}
C = { (4,1) , (3,1) , (3,2) , (2,1) , (2,2) , (2,3) , (1,1) , (1,2) , (1,3) , (1,4)}
(a) \(A\cap B = \phi\)
B & A are mutually exclusive events.
The statement is true.
(b) \(A\cap B = \phi\) and \(A\cup B = S\)
B & A are mutually exclusive and exhaustive events.
The statement is true.
(c) B’ = {(4,1) , (4,2) , (4,3) , (4,4) , (4,5) , (4,6) , (6,1) , (6,2) , (6,3) , (6,4) , (6,5) , (6,6) , (2,1) , (2,2) , (2,3) , (2,4) , (2,5) , (2,6)} = A
The statement is true.
(d) \(A\cap C \) = {(2,1) , (2,2) , (2,3), (4,1)} = \( \phi\)
C and A are not mutually exclusive events.
The statement is false.
(e) \(A\cap B’ = A\cap A = A\)
\(A\cap B \) = \( \phi\)
B’ and A are not mutually exclusive events.
The statement is false.
(f) \(A’\cap B’\cap C\) = S
\(B\cap C \) = { (4,1) , (2,3) , (2,2) , (2,1) } = \( \phi\)
Events B’, C and A’ are not mutually exhaustive and exclusive.
The statement is false.
Exercise 16.3
Question 1
Judge the outcome of the following assignment is valid or not.
S = {\(\omega_{7} ,\omega_{6} ,\omega_{5} ,\omega_{4} ,\omega_{3} ,\omega_{2} ,\omega_{1}\)}
| Assignment | \(\omega_{1}\) | \(\omega_{2}\) | \(\omega_{3}\) | \(\omega_{4}\) | \(\omega_{5}\) | \(\omega_{6}\) | \(\omega_{7}\) |
| (a) | 0.1 | 0.01 | 0.05 | 0.03 | 0.01 | 0.2 | 0.6 |
| (b) | \(\frac{1}{7}\) | \(\frac{1}{7}\) | \(\frac{1}{7}\) | \(\frac{1}{7}\) | \(\frac{1}{7}\) | \(\frac{1}{7}\) | \(\frac{1}{7}\) |
| (c) | 0.1 | 0.2 | 0.3 | 0.4 | 0.5 | 0.6 | 0.7 |
| (d) | -0.1 | 0.2 | 0.3 | 0.4 | -0.2 | 0.1 | 0.3 |
| (e) | \(\frac{1}{14}\) | \(\frac{2}{14}\) | \(\frac{3}{14}\) | \(\frac{4}{14}\) | \(\frac{5}{14}\) | \(\frac{6}{14}\) | \(\frac{15}{14}\) |
Sol: Based on formulae given in Probability
(a)
| ω1 | ω2 | ω3 | ω4 | ω5 | ω6 | ω7 |
| 0.1 | 0.01 | 0.05 | 0.03 | 0.01 | 0.2 | 0.6 |
Each of the numbers such as \(p(\omega_{1}),p(\omega_{2})……..p(\omega_{7})\) are positive in nature and are less than 1.
= \(p(\omega_{7})+p(\omega_{6})+p(\omega_{5})+p(\omega_{4})+ p(\omega_{3})+p(\omega_{2})+p(\omega_{1})\)
= 0.1 + 0.01 + 0.05 + 0.03 + 0.01 + 0.2 + 0.6
= 1
The assignment is valid.
(b)
| ω1 | ω2 | ω3 | ω4 | ω5 | ω6 | ω7 |
| \(\frac{1}{7}\) | \(\frac{1}{7}\) | \(\frac{1}{7}\) | \(\frac{1}{7}\) | \(\frac{1}{7}\) | \(\frac{1}{7}\) | \(\frac{1}{7}\) |
Each of the numbers such as \(p(\omega_{1}),p(\omega {2})……..p(\omega {7})\) are positive in nature and are less than 1.
= \(p(\omega_{7})+p(\omega_{6})+p(\omega_{5})+p(\omega_{4})+ p(\omega_{3})+p(\omega_{2})+p(\omega_{1})\)
= \(\frac{1}{7}\) + \(\frac{1}{7}\) + \(\frac{1}{7}\) + \(\frac{1}{7}\) + \(\frac{1}{7}\) + \(\frac{1}{7}\) + \(\frac{1}{7}\)
= 7 (\(\frac{1}{7}\))
= 1
The assignment is valid.
(c)
| ω1 | ω2 | ω3 | ω4 | ω5 | ω6 | ω7 |
| 0.1 | 0.2 | 0.3 | 0.4 | 0.5 | 0.6 | 0.7 |
Each of the numbers such as \(p(\omega_{1}),p(\omega {2})……..p(\omega {7})\) are positive in nature and are less than 1.
= \(p(\omega_{7})+p(\omega_{6})+p(\omega_{5})+p(\omega_{4})+ p(\omega_{3})+p(\omega_{2})+p(\omega_{1})\)
= 0.1 + 0.2 + 0.3 + 0.4 + 0.5 + 0.6 + 0.7
= 2.8
≠ 1
The assignment is not valid.
(d)
| ω1 | ω2 | ω3 | ω4 | ω5 | ω6 | ω7 |
| -0.1 | 0.2 | 0.3 | 0.4 | -0.2 | 0.1 | 0.3 |
From the above table it is evident that \(p(\omega_{1})\) & \(p(\omega_{5})\) are negative.
Therefore,
The assignment is not valid.
(e)
| ω1 | ω2 | ω3 | ω4 | ω5 | ω6 | ω7 | |||||
| \(\frac{1}{14}\) | \(\frac{2}{14}\) | \(\frac{3}{14}\) | \(\frac{4}{14}\) | \(\frac{5}{14}\) | \(\frac{6}{14}\) | \(\frac{7}{14}\) | |||||
\(p(\omega_{7})\) = \(\frac{15}{14}\) ˃ 1
The assignment is not valid.
Question 2
An experiment consists of tossing up of a coin, determine the probability of getting at least one tail?
Sol: Based on formulae given in Probability
Sample space of the experiment when the coin is tossed twice.
S = {TT , TH , HT , HH }
Let the event P be the event of occurring at least 1 tail.
According to the question = { TT , TH , HT }
Therefore, P (P) = \(\frac{No.\,of\,favourable\,outcomes}{Total\,no.\,of\,outcomes}\)
= \(\frac{n(P)}{n(S)}\)
= \(\frac{3}{4}\)
Question 3
An experiment consists of throwing of a dice in which the following events occurs:
(a) A prime number
(b) Number larger than 3
(c) Number less than or equal to 1
(d) Number more than 6
(e) Number less than 6
Sol: Based on formulae given in Probability
Sample space: { 6 , 5 , 4 , 3 , 2 , 1 }
(a) Let us consider event P be the event of occurrence of prime number
P = { 5 , 3 , 2 }
=\(\frac{No.\,of\,favourable\,outcomes}{Total\,no.\,of\,outcomes}\)
= \(\frac{n(P)}{n(S)}\)
= \(\frac{3}{6}\)
= \(\frac{1}{2}\)
(b) Let us consider event Q be the event of occurrence of a number greater than or equals to 3
Q = { 6,5,4,3}
=\(\frac{No.\,of\,favourable\,outcomes}{Total\,no.\,of\,outcomes}\)
= \(\frac{n(Q)}{n(S)}\)
= \(\frac{4}{6}\)
= \(\frac{2}{3}\)
(c) Let us consider event R be the event of occurrence of a number less than or equals to 1
R = { 1 }
=\(\frac{No.\,of\,favourable\,outcomes}{Total\,no.\,of\,outcomes}\)
= \(\frac{n(R)}{n(S)}\)
= \(\frac{1}{6}\)
(d) Let us consider event S be the event of occurrence of a number greater than 6
S = \(\phi\)
=\(\frac{No.\,of\,favourable\,outcomes}{Total\,no.\,of\,outcomes}\)
= \(\frac{n(S)}{n(S)}\)
= \(\frac{0}{6}\)
= 0
(e) Let us consider event T be the event of occurrence of a number less than 6
T = { 5 , 4 , 3 , 2 , 1 }
=\(\frac{No.\,of\,favourable\,outcomes}{Total\,no.\,of\,outcomes}\)
= \(\frac{n(T)}{n(S)}\)
= \(\frac{5}{6}\)
Question 4
An experiment consists of a pack of 52 cards.
(a) Find the number of elements present in the sample space.
(b) Find the probability of ace of spades
(c) Find the probability of (i) an ace (ii) black card
Sol: Based on formulae given in Probability
(a) When a card is drawn from a deck of cards having a total of 52 cards , the total number of possible outcomes is 52 that i.e 13 cards of each kind ( clubs , spades , diamonds and hearts )
(b) Let us consider P be the event of drawing an ace card .
Number of favourable outcomes = n (P) = 4
P (E) =\(\frac{No.\,of\,favourable\,outcomes}{Total\,no.\,of\,outcomes}\)
= \(\frac{n(P)}{n(S)}\)
= \(\frac{1}{52}\)
= \(\frac{1}{52}\)
(c) Let us consider Q be the event of drawing an ace card.
Number of favourable outcomes = n (Q) = 4
P (E) =\(\frac{No.\,of\,favourable\,outcomes}{Total\,no.\,of\,outcomes}\)
= \(\frac{n(Q)}{n(S)}\)
= \(\frac{4}{52}\)
= \(\frac{1}{13}\)
Consider R be the event of drawing a black card.
Number of favourable outcomes = n (R) = 26
P (E) =\(\frac{No.\,of\,favourable\,outcomes}{Total\,no.\,of\,outcomes}\)
= \(\frac{n(R)}{n(S)}\)
= \(\frac{26}{52}\)
= \(\frac{1}{2}\)
Question 5
An unbiased coin is marked 1 on one face while 6 on the other face , while the die is having markings such as 1,2,3,4,5,6 on its 6 faces .
Determine the probability that the sum of the numbers turning up is
(i) 3
(ii) 12
Sol: Based on formulae given in Probability
According to the question, an unbiased coin is marked 1 on one of the face and 6 on the other face . Also, a die is having 6 faces with marking 1 to 6 respectively.
Sample space (S) = {(1,1) , (1,2) , (1,3) , (1,4) , (1,5) , (1,6) , (6,1) , (6,2) , (6,3) , (6,4) ,(6,5) ,(6,6)}
Therefore = n(s) = 12
(i) Let us consider P be the event of having the sum of the numbers turning up is 3 = P = { (1,2) }
Accordingly, P (E) =\(\frac{No.\,of\,favourable\,outcomes}{Total\,no.\,of\,outcomes}\)
= \(\frac{n(P)}{n(S)}\)
= \(\frac{1}{12}\)
(ii) Let us consider Q be the event of having the sum of the numbers turning up is 12 = P = { (6,6) }
Accordingly, P (E) =\(\frac{No.\,of\,favourable\,outcomes}{Total\,no.\,of\,outcomes}\)
= \(\frac{n(Q)}{n(S)}\)
= \(\frac{1}{12}\)
Question 6
A city council consists of 4 men and 6 women .Find the probability of selecting a woman among the council members if the selection is on random basis.
Sol: Based on formulae given in Probability
According to the question, the city council consists of 4 men and 6 women . Council members are selected on random basis one at a time .
Sample space = 6+4 = 10
Let p be the event of selecting a women among the council members of a city council = n (P) = 6
P (E) = =\(\frac{No.\,of\,favourable\,outcomes}{Total\,no.\,of\,outcomes}\)
= \(\frac{n(P)}{n(S)}\)
= \(\frac{6}{10}\)
= \(\frac{3}{5}\)
Question 7
An experiment consists of tossing up of an unbiased coin 4 times. Each time head appears a person is awarded with Re.1 and each time a tail a turns up that person looses Rs.1.50. Determine sample space of different money of 4 tosses and the propability of each of the head and tail.
Sol: Based on formulae given in Probability
According to the question , the coin is tossed 4 times. Each time head appears the person is awarded with Re.1 but if tails appears that person looses Rs.1.50.
At most 4 heads can turn up = Re. 1 + Re. 1 + Re. 1 + Re. 1
= Rs. 4 (Gain )
When 3 heads and 1 tail appears = Re. 1 + Re. 1 + Re. 1 – Rs.1.50
= Rs. 1.50
When 2 heads and 2 tail appears = Re. 1 + Re. 1 – Rs. 1.50 – Rs.1.50
= -Re. 1.00
When 1 heads and 3 tail appears = Re. 1 – Rs. 1.50 + Rs.1.50 – Rs.1.50
= -Rs. 3.50
At least no head appears , all the 4 times tail appears = – Rs.1.50 – Rs.1.50 – Rs.1.50 – Rs.1.50
= Rs. – 6 (loss )
In the sample space total number of elements = \(2^{4}\) elements
= 16 elements
Sample space (S) = { TTTT , TTTH , TTHT , THTT , HTTT , THHT , THTH , HTHT , TTHH , HTTH , HHTT , THHH , HTHH , HHTH , HHHT , HHHH }
The person gains Rs. 4 for 4 head turn ups for the sample event { HHHH }
Probability ( Gain of Rs. 4 ) = \(\frac{1}{16}\)
The person gains Rs. 1.50 for 3 head turn ups for the sample event { HHHT , HHTH , HTHH , THHH }
Probability ( Gain of Rs. 1.50 ) = \(\frac{4}{16}\) = \(\frac{1}{4}\)
The person looses Re. 1 for 2 head turn ups for the sample event { HHTT , HTTH , TTHH , HTHT , THTH , THHT }
Probability ( Loss of Re. 1 ) = \(\frac{6}{16}\) = \(\frac{3}{8}\)
The person looses Rs. 3.50 for 1 head and 3 tails turn ups for the sample event { TTTT }
Probability ( Loss of Rs. 6 ) = \(\frac{1}{16}\)
Question 8
An experiment consists of tossing up of 3 coins .Find out the probability of the following events:
(a) Three heads
(b) Two heads
(c) At least two heads
(d) At most two heads
(e) No heads
(f) Three tails
(g) Exactly two tails
(h) No tails
(i) At most two tails
Sol:
According to the question , an experiment consists of tossing up of three coins .
Sample space of total number of possible events = { HHH , HHT , HTH , THH , HTT , THT , TTH , TTT }
So, the total number of possible outcomes = n(s) = 8
P (A) = =\(\frac{No.\,of\,favourable\,outcomes}{Total\,no.\,of\,outcomes}\)
(a) Let Q be the event of occurring three heads = { HHH }
Therefore , P(Q) = \(\frac{n(Q)}{n(S)} = \frac{1}{8}\)
(b) Let R be the event of occurring two heads = { HHT , HTH ,THH }
Therefore , P(R) = \(\frac{n(R)}{n(S)} = \frac{3}{18} \)
(c) Let S’ be the event of occurring at least 2 heads = { HHH ,HHT ,HTH ,THH }
Therefore , P(S’) = \(\frac{n(S’)}{n(S)} = \frac{4}{8} = \frac{1}{2}\)
(d) Let T be the event of occurring at most 2 heads = { HHT , HTH ,THH , HTT , THT , TTH , TTT }
Therefore , P(T) = \(\frac{n(T)}{n(S)} = \frac{7}{8}\)
(e) Let U be the event of occurring no heads = { TTT }
Therefore , P(U) = \(\frac{n(U)}{n(S)} = \frac{1}{8}\)
(f) Let V be the event of occurring 3 tails = { TTT }
Therefore , P(V) = \(\frac{n(V)}{n(S)} = \frac{1}{8}\)
(g) Let W be the event of occurring exactly two tails = { HTT , THT , TTH }
Therefore , P(W) = \(\frac{n(W)}{n(S)} = \frac{3}{8}\)
(h) Let X be the event of occurring no tails = { HHH }
Therefore , P(X) = \(\frac{n(X)}{n(S)} = \frac{1}{8}\)
(i) Let Y be the event of occurring at most 2 tails = { HHH , HHT , HTH , THH , HTT , THT , TTH }
Therefore , P(Y) = \(\frac{n(Y)}{n(S)} = \frac{7}{8}\)
Question 9
The probability of an event is \(\frac{2}{11}\) . What is the probability of getting not the above mentioned event.
Sol: Based on formulae given in Probability
Let the event be P and P’
Probability ( P) = \(\frac{2}{11}\)
Probability (P’ ) = 1 – \(\frac{2}{11}\) = \(\frac{9}{11}\)
Question 10
From the word ‘ASSASSINATION’ a letter is chosen at a random basis. Then, determine the probability of getting (a) a vowel (b) an consonant
Sol: Based on formulae given in Probability
Total number of letters in the word ASSASSINATION is 13
Therefore, n(S) = 13
(a) In the above word there are 6 vowels.
Probability (Vowel ) = \(\frac{6}{13}\)
(b) In the above word there are 7 consonants .
Probability ( Consosnant ) = \(\frac{7}{13}\)
Question 11
A person chooses 6 different natural numbers at random basis from numbers ranging from 1 to 20. If and only if this 6-digit number matches with the number decided by the lottery committee , the person wins the prize. Determine the probability of wining the prize ?
Sol: Based on formulae given in Probability
According to the question , total number of ways 6 numbers can be chosen from numbers ranging from 1 to 20
= \(_{6}^{20}\textrm{C}\)
= \(\frac{20 \times 19 \times 18 \times 17 \times 16 \times 15}{1 \times 2 \times 3 \times 4 \times 5 \times 6}\)
= 38760
Therefore the total possibilities are 38760
Out of this 38760 there is only 1 chance to win the lottery,
Probability = \(\frac{1}{38760}\)
Question 12
Check whether the following probabilities are defined or not :
(i) P(Q) = 0.5 , P(R) = 0.7 , P(\(Q \cap R\)) = 0.6
(ii) P(Q) = 0.5 , P(R) = 0.4 , P(\(Q \cup R\)) = 0.8
Sol: Based on formulae given in Probability
(i) P(Q) = 0.5 , P(R) = 0.7 , P(\(A \cap B\)) = 0.6
E and F are two events such that P(E) ≤ P(F)
According to the question ,
P(\(Q \cap R\)) ˃ P(Q)
P(Q) and P(R) are not defined.
(ii) P(Q) = 0.5 , P(R) = 0.4 , P(\(Q \cup R\)) = 0.8
E and F are two events such that P(E) ≤ P(F)
According to the question ,
P(\(Q \cup R\)) ˃ P(Q)
P(Q) and P(R) are defined.
Question 13
| P(Q) | P(R) | \(P(Q\cap R)\) | \(P(Q\cup R)\) | |
| (i) | \(\frac{1}{3}\) | \(\frac{1}{5}\) | \(\frac{1}{15}\) | …. |
| (ii) | 0.35 | …………… | 0.25 | 0.6 |
| (iii) | 0.5 | 0.35 | ……… | 0.7 |
Sol: Based on formulae given in Probability
(i) \(P(Q\cup R) = P(Q) + P(R) – P(Q\cap R )\)
= \(P(Q\cap R ) = \frac{1}{3} + \frac{1}{5} – \frac{1}{15}\)
= \(\frac{7}{15}\)
(ii) \(P(Q\cup R) = P(Q) + P(R) – P(Q\cap R )\)
= 0.6 = 0.35 + P(R) – 0.25
= P(R) = 0.6 – 0.35 +0.25
= P(R) = 0.5
(iii) \(P(Q\cup R) = P(Q) + P(R) – P(Q\cup R )\)
= \(P(Q\cap R ) = 0.5 +0.35 – 0.7\)
= \(P(Q\cap R ) = 0.15\)
Question 14
P(Q) = \(\frac{3}{5}\) and P(R) = \(\frac{1}{5}\). find the value of P( Q or R ) if Q and R are mutually exclusive events.
Sol: Based on formulae given in Probability
According to the question Q and R are mutually exclusive events .
P(Q or R) = P(Q) + P(R)
= P( Q or R ) = \(\frac{3}{5}\) + \(\frac{3}{5}\)
= \(\frac{4}{5}\)
Question 15
If Q and R are events such that P(Q) = \(\frac{1}{4}\) and P(R) = \(\frac{1}{2}\) and P( Q and R) =\(\frac{1}{8}\) . Find the value of P( Q and R )
(a) P( Q or R)
(b) P( not Q and not R)
Sol: Based on formulae given in Probability
(a) P(Q or R) = P(Q) + P(R) – P( Q and R)
= P(Q or R) = \(\frac{1}{4} + \frac{1}{2} – \frac{1}{8}\) = \(\frac{5}{8}\)
(b) P( Q or R) = P(\(Q\cup R\)) = \(\frac{1}{8}\)
= P( not Q and not R) = P(\(Q\cup R\))’
P( not Q and not R) = 1 – P(Q and R)
= P( not Q and not R) = 1 – P(\(Q\cup R\))
= 1 – \(\frac{5}{8}\)
= \(\frac{3}{8}\)
Question 16
Events Q and R are such that P(not Q or not R ) = 0.25 . Find out whether Q and R are mutually exclusive or not ?
Sol:
Given, P ( not Q or not R ) = 0.25
= P(\(Q’ \cup R’\)) = 0.25
= P(\(Q’ \cap R’\)) = 0.25
= P(\(Q\cup R\)) = 1- P(\(Q\cup R\))’
= P(\(Q\cup R\)) = 1- 0.25
= P(\(Q\cup R\)) = 0.75
P(\(Q\cup R\)) ≠ \(\phi\)
Question 17
Events Q and R are such that P(Q) = 0.42 , P® = 0.48 & P(Q and R) = 0.16. Find:
(a) P( not Q)
(b) P(not R)
(c) P( Q or R)
Sol: Based on formulae given in Probability
(a) P(not Q) = 1 – P(Q) = 1 – 0.42 = 0.58
(b) P(not R) = 1 – P(R) = 1 – 0.42 = 0.52
(c) P( Q or R) = P(Q) + P(R) – P( Q and R)
= 0.42 + 0.48 – 0.16 = 0.74
Question 18
In class XII of a school , 40% of the students studies mathematics & 30% of the students studies biology. 10% of the students of the class studies both maths and biology. If a student is selected as a random from the class , determine the probability of the student studying mathematics or biology.
Sol: Based on formulae given in Probability
Let Q be the event in which students studies mathematics and R be the event in which student’s studies biology.
Now,
P(Q) = 40% = \(\frac{40}{100}\) = \(\frac{2}{5}\)
P(R) = 30% = \(\frac{30}{100}\) = \(\frac{3}{10}\)
P(Q and B) = \(\frac{10}{100}\) = \(\frac{1}{10}\)
We know that P( Q or R) = P(Q) + P(R) – P( Q and R)
= P( Q or R) = \(\frac{2}{5}\) + \(\frac{3}{10}\) – \(\frac{1}{10}\)
= 0.6
Therefore , the probability that the students studying mathematics or biology is 0.6
Question 19
In an entrance test that is based on the basis of two examinations, the probability of a random student passing the examination is 0.8 and the probability of the candidate passing the second examination is 0.7. The probability of passing at least one of the examination is 0.95. find out the probability of passing both the examinations?
Sol: Based on formulae given in Probability
Let Q and R be the events of candidates passing the first and second examinations respectively.
Accordingly to the question ,
P( Q or R ) = P(Q) + P(R) – P( Q and R)
= 0.95 = 0.8 + 0.7 – P( Q and R)
= P( Q and R) = 1.5 – 0.95
= P( Q and R) = 0.55
Hence , the probability of the candidates passing both the examinations is 0.55
Question 20
The probability that a student will pass the final examination in oth English and Hindi is 0.5. The probability of passing the neither of the subjects is 0.1. If the probability of passing the subject English alone is 0.75 then, find out the probability of passing in the Hindi subject?
Sol: Based on formulae given in Probability
Let Q and R be the events of passing English and Hindi examinations respectively .
P( Q and R ) =0.5
P( not Q and not R) = 0.1
P(Q) = 0.75
Therefore ,
= ( \(Q\cup R\))’ = (\(Q’\cap R’\))
= P(\(Q\cup R\))’ = P(\(Q’\cap R’\))
= P(\(Q\cup R\)) = 1 – (\(Q\cup R\))’ = 1 – 0.1 = 0.9
We know,
P( Q or R) = P( Q) + P(R) – P( Q and R)
= 0.9 = 0.75 + P(B) – 0.5
= P(B) = 0.65
Therefore the probability of passing the Hindi subject alone in the final examination is 0.65
Question 21
A class of strength 60 students , 30 students opted for NCC, 32 opted for NSS and 24 students opted for both NCC and NSS. Find the probability if one student is selected at a random that:
(a) Candidate opted out for NCC or NSS
(b) Candidates opted neither NCC nor NSS
(c) Candidates opted for NSS but not NCC
Sol: Based on formulae given in Probability
Let Q be the event in which students opted for NCC whereas R be the event in which students in which students opted for NSS
Total strength of the class = 60
Students opted for NCC alone = 30
P( Q) = \(\frac{30}{60} = \frac{1}{2}\)
Students opted for NSS = 32
P(R) = \(\frac{32}{60} = \frac{8}{15}\)
Number of students opted for both NCC and NSS = 24
P( Q and R) = \(\frac{24}{60} = \frac{2}{5}\)
(a) P(Q and R) = P(Q) + P(R) – P( Q and R)
= P(Q and R) = \(\frac{1}{2}\) + \(\frac{8}{15}\) – \(\frac{2}{5}\)
= P(Q and R) = \(\frac{19}{30}\)
Therefore , the students opted for both NCC and NSS is \(\frac{19}{30}\)
(b) P(not Q and not R) = P(A’ and B’)
= P(\(A’\cap B’\))
= P(\(A\cup B\))’
= 1 – P(\(A\cup B\))’
= 1 – P(Q or R)
= 1 – \(\frac{19}{30}\)
= \(\frac{11}{30}\)
Probability that the students opted neither for NCC nor NSS is \(\frac{19}{30}\)
(c) The above mentioned problem can be best visualized with the help of vein diagram.
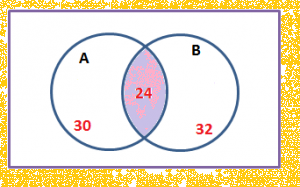
The above diagram shows the number of students who opted for NCC but not NSS
Thus,
= n(R-Q) = n( R) – n(\(A\cap B\))
= 32-24
= 8
Therefore the probability of the students who have opted for NCC but not NSS is
\(\frac{8}{60}\) = \(\frac{2}{15}\)