Examples

Q. The difference between two whole numbers is 66. The ratio of the two numbers is 2 : 5. What are the two numbers?

Ans: Since the ratio of the two numbers is 2 : 5, we may take one number to be 2x and the other to be 5x. (Note that 2x : 5x is same as 2 : 5.) The difference between the two numbers is (5x – 2x). It is given that the difference
is 66. Therefore, 5x – 2x =66 and x=22.
Since the numbers are 2x and 5x, they are 2 × 22 or 44 and 5 × 22 or 110

Q. Deveshi has a total of Rs 590 as currency notes in the denominations of Rs 50, Rs 20 and Rs 10. The ratio of the number of Rs 50 notes and Rs 20 notes is 3:5. If she has a total of 25 notes, how many notes of each denomination she has?

Ans: Let the number of Rs 50 notes and Rs 20 notes be 3x and 5x, respectively.
But she has 25 notes in total. Therefore, the number of Rs 10 notes = 25 – (3x + 5x) = 25 – 8x The amount she has from
Rs. 50 notes : 3x × 50 = Rs 150x from
Rs 20 notes : 5x × 20 = Rs 100x
from Rs 10 notes : (25 – 8x) × 10 = Rs (250 – 80x) Hence the total money she has =150x + 100x + (250 – 80x) = Rs (170x + 250)
But she has Rs 590. Therefore, 170x + 250 = 590 or 170x = 590 – 250 = 340 or x = 2
The number of Rs 50 notes she has = 3x = 3 × 2 = 6
The number of Rs 20 notes she has = 5x = 5 × 2 = 10
The number of Rs 10 notes she has = 25 – 8x = 25 – (8 × 2) = 25 – 16 = 9

Q.If you subtract 1/2 from a number and multiply the result by 1/2 you get 1/8. What is the number ?

Ans : (x - 1/2 ) * 1/2 = 1/8
Therefore x=3-4.

Q.The perimeter of a rectangular swimming pool is 154 m. Its length is 2 m more than twice its breadth. What are the length and the breadth of the pool?

Ans : The breadth be 'x' m and the length we get as '2x+2'm. Perimeter formula is 2(l+b)=154 substituting values of 'l' and 'b' we get x = 25.

Q. Two numbers are in the ratio 5:3. If they differ by 18, what are the numbers?

Ans.

Common ratio is x and so the numbers be 5x and 3x. Difference between them is 18. Solving this we get the numbers.

Q. The ages of Rahul and Haroon are in the ratio 5:7. Four years later the sum of their ages will be 56 years. What are their present ages?

Ans.

The common ratio be 'x' and so the ages are 5x and 7x. So we get 5x+7x+8=56. Solving this we get value of 'x'.

Q. Lakshmi is a cashier in a bank. She has currency notes of denominations Rs. 100, Rs. 50 and Rs. 10, respectively. The ratio of the number of these notes is 2:3:5. The total cash with Lakshmi is Rs. 4,00,000. How many notes of each denomination does she have?

Ans. Let the common ratio be 'x' and so the number of notes be 2x, 3x and 5x. So the amount in Rs. 100 notes is 200x, in Rs. 50 notes is 150x and Rs.10 notes is 50x.
The total amount 400000 = 200x+150x+50x.

Q.I have a total of Rs. 300 in coins of denomination Rs. 1, Rs. 2 and Rs. 5. The number of Rs. 2 coins is 3 times the number of Rs. 5 coins. The total number of coins is 160. How many coins of each denomination are with me?

Ans.Let the Rs. 5 coins be 'x' and so Rs. 2 coins are 3x.
We get Rs. 1 coins as 160-(x+3x).
The amount in Re.1 coins = 160-4x, in Rs.2 coins is 6x and Rs.5 coins is 5x.
Total => 160 = 160 - 4x+6x+5x. We get 'x' is 20.

Q.The organisers of an essay competition decide that a winner in the competition gets a prize of Rs. 100 and a participant who does not win gets a prize of Rs. 25. The total prize money distributed is Rs. 3,000. Find the number of winners, if the total number of participants is 63.

Ans. Let the number of winners be 'x' and so those who couldn't win are 63-x. The winner got Rs. 100x in all. The losers got Rs. 25(63-x) in all.
The equation is:
25(63-x) + 100x = 3000 and so we get 'x' as 19.

Q. The digits of a two-digit number differ by 3. If the digits are interchanged, and the resulting number is added to the original number, we get 143. What can be the original number?

Ans : Let digit in units place be 'b' and so the digit in tens place is 'b+3'. We get the original number as: 10(b+3)+b and the interchanged number as 10b+(b+3).
The sum of both numbers is 143.
10b+(b+3) + 10(b+3)+b = 143. Solving we get b as 5.

Q. A positive number is 5 times another number. If 21 is added to both the numbers, then one of the new numbers becomes twice the other new number. What are the numbers?

Ans :
Let the number be 'x' and 5x. We add 21 to both we get: 2 * (21+x) = 5x+21
So we have x as 7

Q. Aman’s age is three times his son’s age. Ten years ago he was five times his son’s age. Find their present ages.

Ans : Aman's sons age be 'x' and so aman is 3x. Then we have 3x-10 = 5*(x-10). Solving this we get the answer as x = 20.

1. the sum of the measures of the external angles of any polygon is 360°


2. The opposite angles of a parallelogram are of equal measure.


3. The adjacent angles in a parallelogram are supplementary i.e. their sum is 180°.


4. The diagonals of a parallelogram bisect each other


5. The sides of rhombus are all of same length; but not the case with the kite. A rhombus is a quadrilateral with sides of equal length. Since the opposite sides of a rhombus have the same length, it is also a parallelogram. A rhombus has all the properties of a parallelogram and also that of a kite.


6. The diagonals of a rhombus are perpendicular bisectors of one another.


7. The rectangle has opposite sides of equal length and its diagonals bisect each other. The diagonals of a rectangle are of equal length.


8. The diagonals of a square are perpendicular bisectors of each other.


9. Kite: A quadrilateral with exactly two pairs of equal consecutive sides. The diagonals are perpendicular to one another and One of the diagonals bisects the other

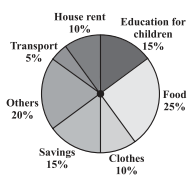
Q.Adjoining pie chart gives the expenditure (in percentage) on various items and savings of a family during a month.
(i) On which item, the expenditure was maximum?
(ii) Expenditure on which item is equal to the total savings of the family?
(iii) If the monthly savings of the family is Rs. 3000, what is the monthly expenditure on clothes?

Ans : (i) Expenditure is maximum on food.
(ii) Expenditure on Education of children is the same (i.e., 15%) as the savings of the family.
(iii)If 15% is Rs. 3000 then 10% is Rs. 2000.

1. if a number has 1 or 9 in the unit’s place, then it’s square ends in 1


2. when a square number ends in 6, the number whose square it is, will have either 4 or 6 in unit’s place.


3. Square numbers can only have even number of zeros at the end


4. There are '2n' non perfect square numbers between the squares of the numbers 'n' and '(n + 1)'.


5. Sum of first n odd natural numbers is n². Also If the number is a square number, it has to be the sum of successive odd numbers starting from 1


6. If a natural number cannot be expressed as a sum of successive odd natural numbers starting with 1, then it is not a perfect square.


7. Consider a number with unit digit 5, i.e., a5. Then (a5)² is = a(a + 1) hundred + 25


8. If a number has unit digit 'a' then the square of the number shall have units digit as the unit digit of a*a. So units digit in square of 81 is 1 as 1*1=1


9. For any natural number m > 1, we have (2m)² + (m² – 1)² = (m² + 1)² . So 2m, m²+1 and m² - 1 forms a Pythagorean triplet with smallest member 2m.


10. If a perfect square is of n-digits, then its square root will have 'n/2' digits if 'n' is even and '(n+1)/2' if 'n' is odd.

1. Consider the following steps to find the square root of 529 and estimate the number of digits in the square root of this number?


2. Step 1 :Place a bar over every pair of digits starting from the digit at one’s place. If the number of digits in it is odd, then the left-most single digit too will have a bar. E.g: Find √ 529 we write it as 5 - 29


3. Step 2 :Find the largest number whose square is less than or equal to the number under the extreme left bar , here (2² < 5 < 3²). Take this number as the divisor and the quotient with the number under the extreme left bar as the dividend (here 5). Divide and get the remainder (1 in this case).


4. Step 3 : Bring down the number under the next bar (i.e., 29 in this case) to the right of the remainder. So the new dividend is 129.


5. Step 4 : Double the quotient and enter it with a blank on its right.


6. Step 5 : Guess a largest possible digit to fill the blank which will also become the new digit in the quotient, such that when the new divisor is multiplied to the new quotient the product is less than or equal to the dividend.
   In this case 42 × 2 = 84.
   As 43 × 3 = 129 so we choose the new digit as 3. Get the remainder.


7. Step 6 : Since the remainder is 0 and no digits are left in the given number, therefore, √529 = 23

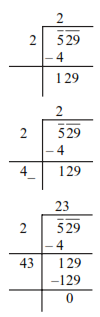
1. If the number has even digits then we can have the same steps. E.g: √4096 can be found by 40 - 96


2. The number of "bar" sign are the number of digits in the square root.


3. For a decimal number like 123.2 we write the bar over the digits before decimal first [right to left] and then after decimal [left to right] and add 0 if needed. So we have 1 - 23 . 20

Q.Find the least number that must be subtracted from 5607 so as to get a perfect square. Also find the square root of the perfect square.

Ans.We put bar over the number. Then the divisor is taken as 7 as 56 lies between 7² and 8². The first division gives 707. Then we double the divisor to 14 and put a 4 next to it as 144*4 = 576. and get 131 as remainder and 74 as quotient.

So 74² is 131 less than 5607. So we subtract 131 from 5607 to get 5476 which is a perfect square.

Q.Find the greatest 4-digit number which is a perfect square.

Ans.We try to find the square root of 9999 by long division. We take first divisor as 9. The second divisor become 189 and remainder is 198. We subtract 198 from 9999 to get 9801 which is a perfect square.

Q. Find the least number that must be added to 1300 so as to get a perfect square. Also find the square root of the perfect square.

Ans.By long division we get 36 as divisor and 4 as remainder and so 36² is less than 1300. 37² is 1369 and so we have to add 69.

Previous

Next