Section 4:Past Years' Solved Questions from the SNAP
4.SNAP 2008
Ans .
(b) Cannot move
- Explanation :
If you look at the chain of forces that the pulley at D puts on the wheel B, there are two forces happening. Through the wheel A, the wheel D is pulling wheel B in an anticlockwise manner. However, through the network of wheels C,E,F,G, the wheel D is pulling the wheel B in a clockwise fashion. Also, since both the forces originate from wheel D, the clockwise and anticlockwise forces on B would be equal. Thus, the wheel would not move. Hence, option (b) is correct.
Ans .
(a) Lo, ba
- Explanation :
Sun Shines brightly = ba lo sul Houses are brightly lit = kado udo ari ba Light comes from sun = dapi kup lo nro. From the first and second statements, we can see that the common word in the original phrases is 'brightly' and the common word in the codes is 'ba'. Thus, we can conclude that brightly means 'ba' in the language. From the first and third statements, we can see that the common word in the original phrases is 'sun' and the common word in the codes is 'lo'. Thus, we can conclude that 'sun' means 'lo' in the language. Thus, option (a) is correct
Ans .
(a) 
- Explanation :
This question has to be solved using the options to confirm how many circles would a square represent so that all the three equations provided to us are consistent with each other, i.e. there is internal consistency between the three equations. Checking option (a) we get: One Square = 5 circles. Putting this value in equation 1: we get: 6 circles = 1 triangle. Thus, equation 3 which shows two triangles on the LHS, would become 12 circles on the LHS and that would be equivalent to 3 diamonds. Thus, 1 diamond would be equal to 4 circles. Putting this value in equation 2 we can see that one square would be equal to 5 circles. Hence, there is internal consistency between the three equations if one square is equivalent to 5 circles. If you check in the same way for the other options, you will see that they would fail the internal consistency check. The equations would not match each other. For instance, if we check option (d) we get: One square = 2 circles. Putting this value in equation 1: we get: 3 circles = 1 triangle. Thus, equation 3, which shows two triangles on the LHS, would become 6 circles on the LHS and that would be equivalent to 3 diamonds. Thus, 1 diamond would be equal to 2 circles. Putting this value in equation 2 we can see that one square would be equal to 3 circles. But we started from 1 square = 2 circles. Hence, internal consistency between the equations is not achieved. Option (d) can be rejected. Checking option (b): One Square = 4 circles. Putting this value in equation 1, we get: 5 circles = 1 triangle. Thus, equation 3, which shows two triangles on the LHS, would become 10 circles on the LHS and that would be equivalent to 3 diamonds. Thus, 1 diamond would be equal to 3.33 circles. Putting this value in equation 2 we can see that one square would be equal to 4.33 circles. But we started from 1 square = 4 circles. Hence, internal consistency between the equations is not achieved. Option (b) can be rejected. Checking option (c): One Square = 3 circles. Putting this value in equation 1: we get: 4 circles = 1 triangle. Thus, equation 3, which shows two triangles on the LHS, would become 8 circles on the LHS and that would be equivalent to 3 diamonds. Thus, 1 diamond would be equal to 2.66 circles. Putting this value in equation 2 we can see that one square would be equal to 3.66 circles. But we started from 1 square = 3 circles. Hence, internal consistency between the equations is not achieved. Option (c) can be rejected. Thus, option (a) is correct.
Ans .
(c) 9
- Explanation :
If the child is a boy, he needs to have at least 4 brothers, means that there are at least 5 boys in the family. By the same reasoning, if the child is a girl, she needs to have at least 3 sisters. This means that there must be at least 4 girls in the family. The minimum number of children the family might have is 5 boys + 4 girls = 9 children. Option (c) is correct.
Ans .
(b) B
- Explanation :
Only conclusion II follows in this case as if some doctors are fools is true it must be true that some fools are doctors. Joshi is a fool is something that is not necessarily true because if he is a doctor, he might or might not belong to the group of doctors who are fools. Thus, option (b) is correct.
Ans .
(d) North-East
- Explanation :
Debu's movements can be tracked as follows:
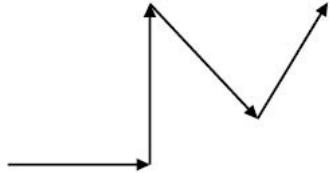 From the figure it is clear that Debu is finally walking towards the North East.
Option (d) is correct.
From the figure it is clear that Debu is finally walking towards the North East.
Option (d) is correct.
Directions for Questions 7 and 9: Read the following instructions and answer. I. There is a rectangular wooden block of length 4 cm, height 3 cm and breadth 3 cm. II. The two opposite surfaces of 4 cm*3 cm are painted yellow on the outside. III. The other two opposite surfaces of 4 cm*3 cm are painted red on the outside. IV. The remaining two surfaces of 3 cm*3 cm are painted green on the outside. V. Now, the block is cut in such a way that cubes of 1 cm*1 cm*1 cm are created.
- Explanation :
The following figure would help you visualise the cube and it's painting.
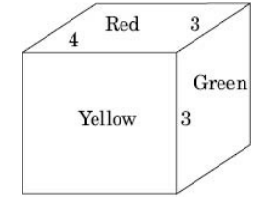 From this visualisation we can visualise the individual surfaces:
The 4 ¥ 3 surfaces would look something like the table below:
From this visualisation we can visualise the individual surfaces:
The 4 ¥ 3 surfaces would look something like the table below:
 We also know that there would be 4 of them.
Similarly, the 3 ¥ 3 surfaces would look something like below and there would be two of
them:
We also know that there would be 4 of them.
Similarly, the 3 ¥ 3 surfaces would look something like below and there would be two of
them:
 From the above visualisations of the surfaces, it is clear that there would be:
2 ¥ 4 + 1 ¥ 2 = 10 cubes with one side painted only.
Option (a) is correct.
From the above visualisations of the surfaces, it is clear that there would be:
2 ¥ 4 + 1 ¥ 2 = 10 cubes with one side painted only.
Option (a) is correct.
- Explanation :
If you look at the front layer of the original cube, there are three such layers. The front layer (where the front is painted yellow); the middle layer and the back layer. If the frontal 4 ¥ 3 layer were to be removed the next layer would have two cubes, which have no color:
 Thus, there would be two cubes with no color
Option (b) is correct.
Thus, there would be two cubes with no color
Option (b) is correct.
- Explanation :
In order to visualise the cubes having two colors we can do so by using: All cubes - cubes having 3 colors - cubes having 1 color - cubes having no color = 36 - 8 - 10 - 2 = 16. Option (c) is correct.
Ans .
(a) 10
Ans .
(b) 2
Ans .
(c) 16
Ans .
(b) B3
- Explanation :
The digits available are 1, 2, 3, 4, 5, 6, 7, 8 and 9. This list has 4 even numbers and 5 odd numbers. Deduction 1 from clue 2: When we know that column A has no odd numbers, we also know that three of the even numbers from 2/4/6 and 8 have to take the places in column A. Deduction 2: C3 - C2 = 4 means that both C3 and C2 contain odd numbers and the pair of numbers they might contain might be: Possibility 1: 9, 5; Possibility 2: 7, 3; Possibility 3: 5, 1 Reading further we know that 7 is in Column B, so we reject the Possibility 2 above and the remaining possibilities for C3 and C2 are 9, 5 and 5,1 respectively. If you combine this information with the fact that Column C adds up to 14, we see that the 9, 5 possibility for Column C does not exist. Thus, the three numbers in the order C1, C2, C3 for Column C are: 8, 5, 1 This also then means that the digits in the first column (A) are 2, 4 and 6, but not necessarily in that order. With these deductions the grid would become:
 Now since we know that the sum of digits in Row 1 is 17, we can definitely conclude that 9
would not be in the first row of Column B. Further, the digit 2 is not in the same row as 8. The
grid would become:
Now since we know that the sum of digits in Row 1 is 17, we can definitely conclude that 9
would not be in the first row of Column B. Further, the digit 2 is not in the same row as 8. The
grid would become:
 Now since 9, is not immediately below 3, 9 would necessarily take the B3 cell.
Option (b) is the correct answer
Now since 9, is not immediately below 3, 9 would necessarily take the B3 cell.
Option (b) is the correct answer
Ans .
(b) 2880
- Explanation :
The logic of the series is 4, 4 ¥ 8 = 32, 32 ¥ 9 = 288, 288 ¥ 10 = 2880, 2880 ¥ 11 = 31680. Thus, the missing number is 2880. Option (b) is correct.
Ans .
(a) '10.50
- Explanation :
The key to the start of the thinking of this question is that the same number of lemons are left at the point of time when Seema is called away. In order to understand the mathematical situation, suppose we assume that there were 30 lemons left with both women at this point of time. If they sold the lemons at their original rates, Jamuna would earn '7.5 while Seema would have earned '5. A total realization of '12.5 By selling the 60 lemons @ 5 lemons per rupee, the net realization for both would be '12. This would mean a loss of '0.5. Since, the loss is '3.5, it means that the number of lemons should be 7 times for both people. Thus, they have 210 lemons each. Total realization = '84. If the money is split half way, Jamuna would have earned '42. Jamuna's realization on her own would be = 7.5 ¥ 7 = 52.5 Thus, Jamuna ends up losing '10.5. Option (a) is correct.
Ans .
(c) Equal amount of each juice between the two cups
- Explanation :
AscendThere would be equal amount of each juice in each cup and this can be experimentally verified. If you were to suppose there were 100 ml in each cup to begin with and let's say we are transferring 10 ml from one cup to the other and back.
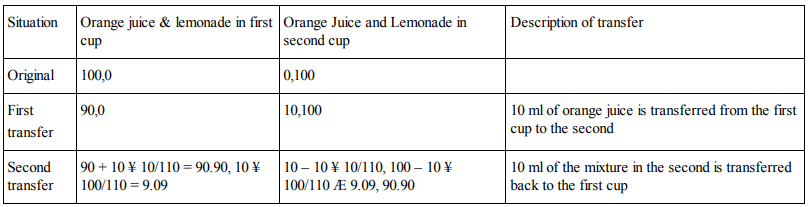 Thus, option (c) is correct
Thus, option (c) is correct
Ans .
(a) Only A is implicit.
- Explanation :
Only A is an implicit assumption as the statement directly connects education and small family norm to the nation's progress. Option (a) is correct.
Ans .
(d) MS,DN
- Explanation :
The two words are Indecent and Immodest. Thus, MS should fill the blanks of the first figure and DN should fill the second figure. Option (d) is correct.
Ans .
(c) During warm weather my dog suffers more fleas than during cool weather. Therefore, fleas must thrive in a warm environment.
- Explanation :
The argument in the question is based on the premise that just because the city's beaches are the most overcrowded in the state, so it must be true that beautiful beaches attract people. The reasoning is akin to making a generic conclusion about something based on one observation. Similar reasoning is shown by option (c) because it uses a similar kind of logic to make a conclusion. On one fact, the whole conclusion is based, viz: just because my dog suffers more fleas during hot weather, it follows that fleas must thrive in a warm environment. Option (c) is correct.
Ans .
(b) 5 yellow birds and 2 non-yellow birds
- Explanation :
This can be put into a grid as follows and in fact becomes much easier to execute inside a grid: Let us say we take 10 birds to start with and we try to see how many are required at the minimum to fulfill all conditions: Let the birds be A,B,C,D,E,F,G,H,I and J. If we meet all of Abdul's requirements, the table would look as below.
 From this point we need to think of how Mala's constraints can be met. If we think of C and G
as yellow birds, we will get Mala having seen 3 yellow birds (A, C and G). Besides, at this
stage we have also taken care of Abdul's constraints. The table would look as below.
From this point we need to think of how Mala's constraints can be met. If we think of C and G
as yellow birds, we will get Mala having seen 3 yellow birds (A, C and G). Besides, at this
stage we have also taken care of Abdul's constraints. The table would look as below. 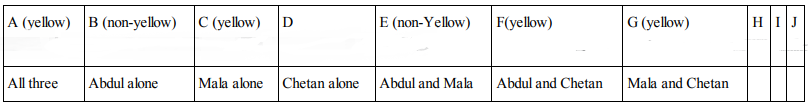 Into this scheme of things, if we now try to put Chetan's constraints into the table we have that
since Chetan has seen at least 4 yellow birds, bird D can be put as yellow. In such a case the
grid would look as below:
Into this scheme of things, if we now try to put Chetan's constraints into the table we have that
since Chetan has seen at least 4 yellow birds, bird D can be put as yellow. In such a case the
grid would look as below:
 This situation meets all the constraints in the problem. Birds H, I and J are not required to be
considered.
There are 5 yellow birds and 2 non-yellow birds.
Option (b) is correct.
This situation meets all the constraints in the problem. Birds H, I and J are not required to be
considered.
There are 5 yellow birds and 2 non-yellow birds.
Option (b) is correct.
Ans .
(a) I-J, II-J
- Explanation :
A lake is still water, the others are names of flowing water bodies. In the second list Weighty-Heavy is the odd pair between the options we have of broad-wide versus weighty-heavy. Option (a) is correct.
Ans .
(d) B & C occupy diagonally opposite positions.
- Explanation :
The final arrangement after the walks would be as shown below:
 It can be clearly seen that B and C are diagonally opposite. Option (d) is correct.
It can be clearly seen that B and C are diagonally opposite. Option (d) is correct.
Ans .
(c) Wish Bones came in first. Sundae and Hallelujah tied for second place. Penn Fe came in fourth. Night Marvel came in fifth.
- Explanation :
The correct order that satisfies all conditions can be checked from the options. Option (a) is incorrect because it contradicts the clue "Penn Fe finished as many places after Sundae as Sundae finished after Wish Bones" which has to be true because then "Wish Bones finish before Night Marvel". Option (b) is incorrect, because it contradicts the same clue, i.e. "Penn Fe finished as many places after Sundae as Sundae finished after Wish Bones" which has to be true because then "Wish Bones finished before Night Marvel". Option (d) is also wrong because in that option, Penn Fe finishes before Wish Bones-which contradicts the first clue that Penn Fe finishes after Wish Bones. Option (c) is correct as it satisfies all the conditions.
Ans .
(b) 20
- Explanation :
If the 6th and the 16th are diametrically opposite, it means that between them on one side there would be 7, 8, 9, 10, 11, 12, 13, 14 and 15, which means there are 9 people on either side. Thus, the total number of people is 20.
Ans .
(b) 72
- Explanation :
The logic for each column is fixed: So the first column has 39 at the bottom because (8 + 5) ¥ 3 = 39. Similarly, in the second column we have: (4 + 7) ¥ 4 = 44. In the third column we have: (9 + 3) ¥ 5 = 60. In the fourth column we would get: (5 + 4) ¥ 8 = 72. Option (b) is correct.
Ans .
(c) C
- Explanation :
You have to visualise the rotation of each cylinder when the cyinders on top push the cylinders below to the sides. Each cylinder would roll by 180 0 and hence, option (c) is correct.
Directions for Questions 24 and 26: Use the information given below to answer. i. There is a group of 5 persons A, B, C, D and E. ii. In the group there is one badminton player, one chess player and one tennis player. iii. A and D are unmarried ladies and they do not play any games. iv. No lady is a chess player or a badminton player. v. There is a married couple in the group of which E is the husband. vi. B is the brother of C and is neither a chess player nor a tennis player.
- Explanation :
The following chain of deductions would give us the solution to this question set. Starting grid:
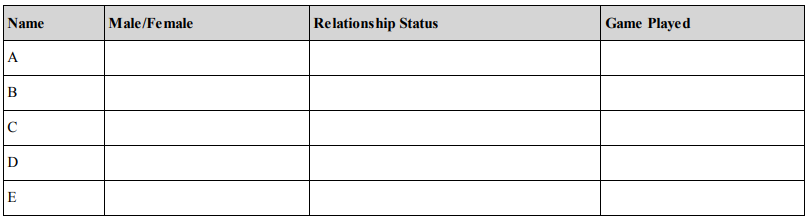 A and D being unmarried ladies who do not play any game, can be put into the grid as
follows:
A and D being unmarried ladies who do not play any game, can be put into the grid as
follows:
 Note: In the above we have concluded that B, C and E have to each play a game as A and D are not
playing any game means that the other three people must be playing a game each.
At this point if we use the information given in clues (v) and (vi) we realise that E and B are
males and since there is a married couple in the group there has to be a married lady and that
must be C. Also since no lady plays chess or badminton it follows that C must play Tennis.
The grid changes to:
Note: In the above we have concluded that B, C and E have to each play a game as A and D are not
playing any game means that the other three people must be playing a game each.
At this point if we use the information given in clues (v) and (vi) we realise that E and B are
males and since there is a married couple in the group there has to be a married lady and that
must be C. Also since no lady plays chess or badminton it follows that C must play Tennis.
The grid changes to:
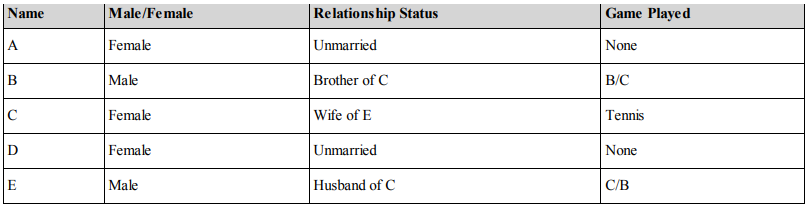 Further, since we know that B is not a chess player, he must be a badminton player. The final
pieces fall into place inside the grid.
Further, since we know that B is not a chess player, he must be a badminton player. The final
pieces fall into place inside the grid.
 The answers to the questions can now be read off the above grid.
ACD is the group of ladies. Option (d) is correct.
The answers to the questions can now be read off the above grid.
ACD is the group of ladies. Option (d) is correct.
- Explanation :
The following chain of deductions would give us the solution to this question set. Starting grid:
 A and D being unmarried ladies who do not play any game, can be put into the grid as
follows:
A and D being unmarried ladies who do not play any game, can be put into the grid as
follows:
 Note: In the above we have concluded that B, C and E have to each play a game as A and D are not
playing any game means that the other three people must be playing a game each.
At this point if we use the information given in clues (v) and (vi) we realise that E and B are
males and since there is a married couple in the group there has to be a married lady and that
must be C. Also since no lady plays chess or badminton it follows that C must play Tennis.
The grid changes to:
Note: In the above we have concluded that B, C and E have to each play a game as A and D are not
playing any game means that the other three people must be playing a game each.
At this point if we use the information given in clues (v) and (vi) we realise that E and B are
males and since there is a married couple in the group there has to be a married lady and that
must be C. Also since no lady plays chess or badminton it follows that C must play Tennis.
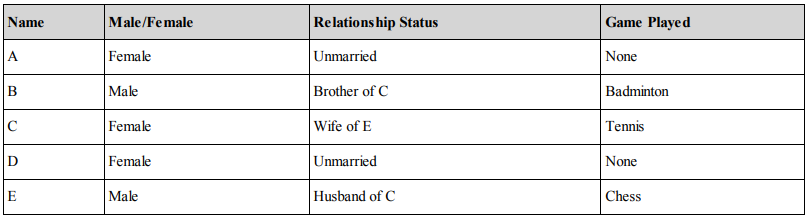
The grid changes to:
 Further, since we know that B is not a chess player, he must be a badminton player. The final
pieces fall into place inside the grid.
Further, since we know that B is not a chess player, he must be a badminton player. The final
pieces fall into place inside the grid.
 The answers to the questions can now be read off the above grid.
C is the tennis player. Option (b) is correct
The answers to the questions can now be read off the above grid.
C is the tennis player. Option (b) is correct - Explanation :
The following chain of deductions would give us the solution to this question set. Starting grid:
 A and D being unmarried ladies who do not play any game, can be put into the grid as
follows:
A and D being unmarried ladies who do not play any game, can be put into the grid as
follows:
 Note: In the above we have concluded that B, C and E have to each play a game as A and D are not
playing any game means that the other three people must be playing a game each.
At this point if we use the information given in clues (v) and (vi) we realise that E and B are
males and since there is a married couple in the group there has to be a married lady and that
must be C. Also since no lady plays chess or badminton it follows that C must play Tennis.
The grid changes to:
Note: In the above we have concluded that B, C and E have to each play a game as A and D are not
playing any game means that the other three people must be playing a game each.
At this point if we use the information given in clues (v) and (vi) we realise that E and B are
males and since there is a married couple in the group there has to be a married lady and that
must be C. Also since no lady plays chess or badminton it follows that C must play Tennis.
The grid changes to:
 Further, since we know that B is not a chess player, he must be a badminton player. The final
pieces fall into place inside the grid.
Further, since we know that B is not a chess player, he must be a badminton player. The final
pieces fall into place inside the grid.
 The answers to the questions can now be read off the above grid.
C is the wife of E. Option (d) is correct.
The answers to the questions can now be read off the above grid.
C is the wife of E. Option (d) is correct.
- Explanation :
The family tree would look like:
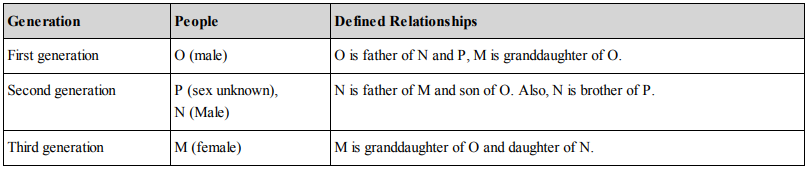 Based on this tree:
Option (a): P is the father of M, contradicts the given premises.
Option (b): O has three children can happen as there could be one more sibing for N and P.
Thus, option (b) does not contradict the premises.
Option (c): Again, this can happen and hence this option does not contradict the premises.
Option (d): is definitely true and does not contradict the premises.
Thus, the statement in option (a) contradicts the premises.
Option (a) is the correct answer.
Based on this tree:
Option (a): P is the father of M, contradicts the given premises.
Option (b): O has three children can happen as there could be one more sibing for N and P.
Thus, option (b) does not contradict the premises.
Option (c): Again, this can happen and hence this option does not contradict the premises.
Option (d): is definitely true and does not contradict the premises.
Thus, the statement in option (a) contradicts the premises.
Option (a) is the correct answer.
Ans .
(d) None of the above
Ans .
(b) C
Ans .
(d) None of the above
Ans .
(a) P is the father of M.
Ans .
(c) C
- Explanation :
On a hot day wind would flow from land to sea. Option (c) is correct
Directions for Questions 29 and 30: In the diagram below, the circle stands for 'educated', square
stands for 'hard working', triangle for 'urban people' and rectangle for 'honest'. The different regions
of the diagram are numbered from 1 to 12. Study the diagram carefully and answer the questions:
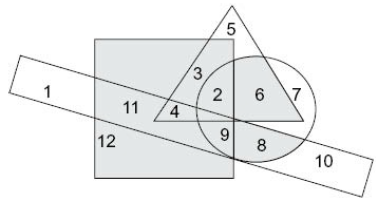
- Explanation :
The required area we are looking for should be outside the circle (for 'uneducated') but inside the triangle (urban), inside the square (hard working) and inside the diagonal rectangle (honest). Area represented by the digit 4 satisfies this condition. Option (d) is the correct answer.
- Explanation :
Non-urban (outside the urban triangle); educated (inside the educated circle); not hardworking (outside the square) and not honest (outside the diagonal rectangle). Area 7 is outside the triangle, the square and the rectangle but inside the circle. Hence, option (b) is the correct answer.
Ans .
(d) 4
Ans .
(b) 7